( Class 12th Physics Chapter 12 Atoms Notes ) www.free-education.in is a platform where you can get pdf notes from 6th to 12th class notes, General Knowledge post, Engineering post, Career Guidelines , English Speaking Trick , How to crack interview and lots more.

Class 12th Physics Chapter 12 Atoms Notes

INTRODUCTION:

Atomis the fundamental building block of matterhaving a confined positively charged nucleus at the center, surrounded by negatively charged electrons.Every inorganic, organic, or even synthetic object is made up of atoms.

There were many atomic models given initially

DALTON’S ATOMIC THEORY:

• Atoms are the smallest constituents of matter and can’t be divided further(indivisible)
• Atoms belonging to same matter have similar characteristics and mass; atoms of different matter have different properties and mass
• Atoms are reoriented in a chemical reaction (not generated or destructed)

Merits:

• It demonstrated laws of: a)mass conservation, b)fixed composition, c)multiple proportions

Demerits:

• It was unable to demonstrate experiments of electrostatics (dry paper bits sticking to comb) that showed charge exists.

THOMSON MODEL OF ATOM:

• Atom is like a sphere where positive charge has uniform distribution throughout
• Electrons are scattered inside in a way that  most stable electrostatic arrangement is achieved, meaning that minimum possible energy of the system should is achieved
• Also known as watermelon model or plum pudding model or raisin bread model
• It positively illustrated the net neutrality(equal positive and negative charges, so no net charge) of atom
• It was inconsistent with the experiments conducted later and the discovery of neutron and proton.

THOMSON’S ATOMIC MODEL

RUTHERFORD’S NUCLEAR MODEL OF ATOM:

• Geiger and Marsden carried out few experiments on the advice of Rutherford
• On a very thin gold foil, highly energetic ray of α-particles(that are positively charged with energy of 5.5MeV)was incident
• Scattered α-particles when strike zinc sulphide screen(surrounding the thin gold foil) produced light flashes(scintillations) which were observed via detector(microscope)

• Scattered α-particles’ distribution as a function of scattering angle(θ) was analyzed and plotted as a graph

• As the scattering angle(θ) got higher, count of scattered α-particles observed got lower, and vice versa
• Almost all of the α-particles passed through the gold foil undeflected, and infinitesimally small number of α-particles got deflected
• This proved that almost all the space in an atom is empty (9999999999996%), and the positive charge is confined to an extremely small region because very fewof the positively charged α-particles were repelled by gold foil proving positive charge is confined to a small region. This region was called nucleus.
• Atom has a structure analogous to our solar system where nucleus (like sun) is at center and all the electrons (like planets) revolving around it in a specified circular path at large speeds

• Electrons and protons are bound together by electrostatic forces of attraction and atom, as a whole is electrically neutral
• Rutherford was the first to discover that atom has a nucleus and so his model was called as Rutherford’s nuclear model of atom
• Electron Orbit:

Since electrons orbiting around the nucleus and are held to nucleus by electrostatic force of attraction, ergo, centripetal force (F_c) is provided by the electrostatic force (F_e) to keep electrons in the orbit.

F_c  = F_e

Here r=radius of orbit, v= velocity of orbiting electron, e= charge of an electron, m= mass of an electron, Z=atomic mass of atom, Ɛ_o=permittivity of free space

On solving, we get:

• Kinetic Energy(K): putting the value of mv²from eq.(1), we get:

• Potential Energy(U): using the electrostatic potential between 2 charged body, we get:

• Negative sign here shows that there is a force of attraction and energy has to be given to the system to overcome this force of attraction
• Total Energy(T):

T = U + K

• Some important things to note:

Kinetic energy(K) = -(1/2)Potential energy(U)

Kinetic energy(K) = -Total energy(T)

Potential energy(U) = 2×Total energy(T)

Drawbacks of Rutherford’s Model:

• Accelerated charged particle produces electromagnetic waves (Maxwell Theory), so orbital radius of electron should go on decreasing and finally electron should fall into the nucleus. But atoms are stable in nature and this stability of atoms could not be clarified by Rutherford’s model
• The model didn’t talk about electronic structure of atoms, viz. electrons orientation, their orbital motion and relative energy of electron in different orbits
• Dual character of electromagnetic radiation couldn’t be elaborated by the model

Numerical Problems: Hydrogen atom has ground state energy of -13.6eV. What are the kinetic and potential energies of atom at this state?

Solution: Given: Total energy= -13.6eV

We know from the above equations: Kinetic energy(K)= -Total energy(T)

∴ K = -(-13.6eV) = 13.6eV (ans)

Potential energy(U) = 2×Total energy(T)

∴ U = 2×(-13.6eV) = -27.2eV (ans)

Note: Here negative sign shows that there is a force of attraction between electron and nucleus.

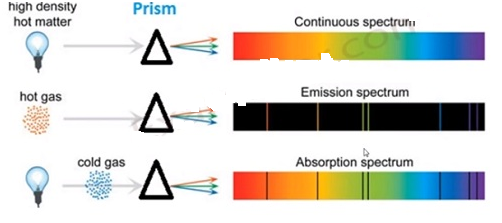
ATOMIC SPECTRA:

• When an electron jumps amongst energy levels in an atom, energy is emitted or absorbed in the form of electromagnetic radiations and these radiations produce a spectral lines of frequencies(or wavelength) associated with an atom, called atomic spectra
• Spectroscopy is the learning and examination emission and absorption spectra associated with an atom to determine its properties
• Spectral lines are the bright and dark line series that constitute the spectrum associated with an atom
• An atom has a discrete spectra(where exist a fixed specific lines of energy transition of electron with discrete energy gaps) also known as quantized spectra
• Another type is continuous spectra(where there is no specific lines of energy transition of electrons) which is the reverse of discrete spectra

• There are 3 types of atomic spectra: a)emission spectra, b)absorption spectra, c) continuous spectra

Emission spectra:

• Radiation spectrum produced due to absorption of energy by a matter
• When an electron of an atom, molecules or ions get to a higher energy state than their ground (stable) state due to radiation absorption, they are said to be excited
• Emission spectrum is produced when energy is supplied to a sample (through heating or irradiation) and the wavelength or frequency of radiation emitted by the sample is observed as a function of energy

Absorption spectra:

• It is just the opposite of emission spectra
• Continuous radiation (energy) is directed through a sample which absorbs certain radiation of particular wavelengths and the remaining spectrum is recorded. Absorbed wavelengths correspond to the dark spaces in the spectrum
• Whatever absent(showed by dark lines) in the emission spectrum of an atom is actually present (showed by bright lines) in the absorption spectrum of that atom

Continuous spectra:

• Formed when a ray of white light is passed through a prism(or water droplets) causing a continuous spectrum of visible light of different wavelength
• There are no discrete lines (separation) between any 2 adjacent wavelengths
• Speed of light changes with respect to the medium through which it passes, so as the medium changes, light with the longest wavelength (red)deviates the least and the light with the shortest wavelength (violet) deviates the most

SPECTRAL SERIES:

• On passing electric discharge through hydrogen gas, hydrogen molecules would dissociate giving rise to excited (highly energetic) hydrogen atoms that emit radiation of certain specified frequency while returning to its ground state
• Hydrogen spectra is constituted of 5 series of spectrum named after their discoverer(Lyman, Balmer, Paschen, Bracket and Pfund series)

TYPES OF SPECTRAL SERIES:

Balmer Series:

• First scientist to discover a spectral series of hydrogen atom
• It consist of visible radiation spectrum
• Experimentally, he found that these spectral lines could be expressed mathematically in the form of wavelength as:

• Here R= Rhydberg constant = 109677cm^-1(found experimentally), n= 3, 4, 5…… (higher discrete energy state from which electron jumps to 2^nd energy state thus emitting radiation)

         λ = wavelength of emitted radiation in (cm)

• For maximum wavelength(λ_max) in the Balmer series, n=3 (has to be minimum):

• For minimum wavelength (λ_min) in the Balmer series, n=∞(has to be minimum):

Lyman Series:

• Spectral series when radiation emitted is due to jumping of electron from higher energy states to ground state
• Mathematically expressed as

• Here n= 2, 3, 4…
• For maximum wavelength  in the Lyman series, n=2 (has to be minimum):

λ_max = 4/3R

• For minimum wavelength in the Lyman series, n=∞(has to be minimum):

λ_min = 1/R

Similarly, all other series could be expressed as:

Paschen Series:

• Mathematically expressed as

• Here n= 4, 5, 6…

Bracket Series:

• Mathematically expressed as

• Here n= 5, 6, 7…

PfundSeries:

• Mathematically expressed as

• Here n= 6, 7, 8…

• Atomic spectra has a huge scope in the study of electronic structures of various atoms, molecules and ions
• Elements have their own distinctive spectral series. So spectral series is used in the identification (even discovery) of unknown atoms
• Many elements were discovered by the spectroscopic methods, such as Rubidium(Ru), Caesium(Cs), Helium(He), Gallium(Ga), Thallium(Tl), Scandium(Sc).

Numerical Problem:Evaluate the shortest and the longest wavelength corresponding to the following series of spectral lines:

1. Lyman series
2. Paschen series
3. Bracket series

Solution:

1. For Lyman series: 1/λ = R(1/1² – 1/n²)

For shortest wavelength (λ_min), n has to be maximum

1/λ_max = R(1/1² – 1/∞²)

λ_max = 1/R = (1/109677)cm

𝜆_min = 9.118×10^-6cm (Answer)

For longest wavelength (λ_max), n has to be minimum

1/λ_max = R(1/1² – 1/2²)

𝜆_max = 4/(3R) = 4/(3×109677) = 1.216×10^-5cm

λ_max = 1.216×10^-5cm (Answer)

1. b) For Paschen series, similarly proceeding as above

For shortest wavelength , n has to be maximum

1/λ_min = R(1/3² – 1/∞²)

𝜆_min = 9/R = 9/109677 = 8.206×10^-5cm (ans)

For longest wavelength , n has to be minimum

1/λ_max = R(1/3² – 1/4²)

λ_max = 16×9/7 =16×9/106977 = 1.876×10^-4cm (Answer)

c) For Bracket series, similarly proceeding as above

1/λ_min = R(1/4² – 1/∞²)

𝜆_min = 16/R = 16/109677 =1.459×10^-4cm

For longest wavelength (λ_max), n has to be minimum

∴1/λ_max = R(1/4² – 1/5²)

𝜆_max= 25×16/(9R) = 4.052×10^-4cm (Answer)

Numerical Problem:A hydrogen atom on absorbing a photon, gets excited to the n=4 level from its ground level. What is the wavelength and frequency of the photon?

Solution: Given, n =4

From Balmer series, we can write
1/𝜆 = R(1/2² – 1/4²) = 109677(1/4 – 1/16)

λ = 16/(3×109677) = 4.86×10^-5cm (ans)

For frequency(υ), we know that it is given by the formula

υ =c/𝜆 = 3×10⁸/(4.86×10^-7) = 6.1×10¹⁴Hz (ans)

Energy of Orbits:

The orbital energy of orbiting electron in the discrete energy levels in the Bohr’s model is called as the energy of orbits.

We already know, from Rutherford’s Model that total energy(T) is given by

T = -Ze²/(8πƐ_or)

Putting the value of Bohr’s radius, we get

• Putting the values of electron mass(m), charge(e), permittivity of free space(Ɛ_o), Planck’s constant(h); we get

T = (-13.6/n²)eV

• For the energy of innermost stationary orbit, put n=1

DRAWBACKS OF BOHR’S MODEL:

• It was primarily for hydrogen atom
• It couldn’t elaborate spectra of multi-electron atoms
• Wave nature of electron was not justified by the model (inconsistent with the de Broglie’s hypothesis of dual nature of matter)
• It didn’t illustrated molecules making process of chemical reactions
• It violated Heisenberg’s Principal(Δx× Δp ≥nh/(2π)) which said that it was impossible to evaluate theprecise position and momentum of electron (and other microscopic particles) simultaneously.Only their probability could be estimated.
• Zeeman effect(spectral lines variation due to external magnetic field) and Stark Effect(spectral lines variation due to external electric field) couldn’t be described by the model

Numerical Problem:The energy gap between the 2 energy levels is 2.3eV. What is the frequency of radiation emitted when the atom makes a transition from higher to lower energy levels?

Solution: Given, ΔE = 2.3eV

Using Bohr’s 2^nd postulate

ΔE = hv

Numerical Problem: Use the Bohr’s model to calculate:

1. Electron speed in the n=1, 2, and 3 levels of the hydrogen atom
2. Bohr’s radius of orbit in each of these levels.

Solution:

1. Velocity of orbiting electron as per Bohr’s model is given by

v = e²/(2nhƐ_o)

For n = 1, velocity is given by

For n = 2, velocity is given by

For n =3, velocity is given by

1. Bohr’s radius of orbit is given by

r = n²h²Ɛ_o/(πme²)

For n = 1, Bohr’s radius will be

For n = 2, Bohr’s radius will be

For n = 3, Bohr’s radius will be

Numerical Problem: Using Bohr’s Model, find the quantum number associated with earth’s revolution around the sun in an orbit of radius5×10¹¹m, orbital speed of3×10⁴m/s, and

mass of earth=6×10²⁴kg

Solution:

Given,  r_n =1.5×10¹¹m, v_n = 3×10⁴m/s, and  m = 6×10²⁴kg

According to the Bohr’s second postulate:

mv_nr_n = nh/(2π)

DE-BROGLIE’S HYPOTHESIS:

• De Broglie’s Hypothesis showed the wave particle duality of matter
• It showed that, like photons, electrons must also have mass or momentum() and wavelength(λ), given by the equation (here c= speed of light in air, v=frequency)

p = mv = h/λ = h/(c/λ)

• It holds only for the subatomic (microscopic) particles like electron, proton etc. where mass is very small, so wavelength are large enough to be experimentally observable
• It does not hold for the macroscopic particles since mass there is very large, making wavelength too small to be experimentally observable

DE-BROGLIE’S EXPLANATION OF  BOHR’S SECOND POSTULATE OF QUANTISATION:

• Electron orbiting in circular orbit can be considered as a particle wave
• Only those waves propagate and survive which form nodes at terminal point with integer multiple of wavelength(resonant standing waves), thus covering the whole circumferential distance of circular orbit

2πr_n = nλ

• From de Broglie’s hypothesis: λ = h/(mv)
• So, ultimately we get: mv_nr_n = nh/(2π)
• This proved that wave-particle duality is the cause of quantized energy states

Chapter-12-Atoms

CBSE Class 12 Physics Important Questions Chapter 12 – Atoms

1.All elements consists of very small invisible particles, called Every atom is a sphere of radius of the order of 10^_10m, in which entire mass is uniformly distributed and negative charged electrons revolve around the nucleus.

3.Impact parameter perpendicular distance of the velocity vector of a-particle from the central line of the nucleus of the atom is called impact parameter (b).

4.Basic assumption of Rutherford’s atomic model

• Atom consists of small central core, called atomic nucleus in which whole mass and positive charge is assumed to be concentrated.
• The size of nucleus is much smaller than the size of the atom.
•  The nucleus is surrounded by electrons and atom is electrically neutral

6.Angle of Scattering Angle by which a-particle gets deviated from its original path around the nucleus is called angle of scattering.

7.Drawbacks of Rutherford’s Model

(i) Could not explained stability of atom clearly.

(ii) Unable to explain line spectrum

11.Energy Level The energy of an atom is the least when its electron is revolving in an orbit closest to the nucleus i.e. for which n = 1.

12.The lowest state of the atom is called the ground state, this state has lowest energy. The energy of this state is -13.6 eV. Therefore, the minimum energy required to free the electron from the ground state of the hydrogen atom is -13.6 eV.

13.(i)Emission Spectrum Hydrogen spectrum consists of discrete bright lines a dark background and it is specifically known as hydrogen emission spectrum.

(ii) Absorption Spectrum There is one more type of hydrogen spectrum exists where we get dark lines on the bright background, it is known as absorption spectrum

Previous Years Examination Questions

1 Mark Questions

1.The ground state energy of hydrogen atom is – 13.6 eV. What are the kinetic and potential energies of electron in this state? [All India 2014C; HOTS; All India 2010]

Ans. Given, total ground state energy (TE) = (-13.6eV)

.-. Kinetic energy = – TE

= -(-13.6 eV) =13.6 eV Potential energy = 2 (TE)

= 2 x(-13.6) = -27.2 eV

2.When is H_a-line of the Balmer series in the emission spectrum of hydrogen atom obtained? [Delhi 2013C]

Ans. H_a-line of the Balmer series in the emission spectrum of hydrogen atom is obtained in visible region.

3.Why is the classical (Rutherford) model for an atom of electron orbitting around the nucleus not able to explain the atomic Structure?  [Delhi 2012]

Ans.  The classical method could not explain the atomic structure as the electron revolving around the nucleus are accelerated and emits energy as the result, the radius of the circular paths goes on decreasing. Ultimately electrons fall into the nucleus, which is not in practical.

4.Define ionisation energy. What is its value for a hydrogen atoms?  [All India 2010]

Ans. Ionisation energy The minimum amount of energy required to remove an electron from the ground state of the atom is known as ionisation energy.

5.Find the ratio of energies of photons produced due to transition of an electron of hydrogen atom from its

• second permitted energy level to the first permitted level and
• the highest permitted energy level to the first permitted level. [All India 2010]

Ans.

6.What is the ratio of radii of the orbits corresponding to first excited state and ground state, in a hydrogen atom?   [Delhi 2010]

Ans.

7.The radius of innermost electron orbit of a hydrogen atom is 5.3x 10-¹¹ What is the radius of orbit in the second excited state?

Ans.

8.Write the expression for Bohr’s radius in hydrogen atom. [Delhi 2010]

Ans.

9.State Bohr’s quantisation condition for defining stationary orbits. [Foreign 2010]

Ans.

10.In the Rutherford scattering experiment, the distance of closest approach for an a-particle is do. If a-particle is replaced by a proton, then how much kinetic energy in comparison to a-particle will be required to have the same distance of Closest approach do ?      [Foreign 2009]

Ans.

---

NCERT Exercises ( Atoms Notes )

---

Question 1. ( Atoms Notes )
Choose the correct alternative from the clues given at the end of each statement:
(a) The size of the atom in Thomson’s model is _____ the atomic size in Rutherford’s model. (Much greater than / no different from / much less than).
(b) In the ground state of _____ electrons are in stable equilibrium, while in _____ electrons always experience a net force. (Thomson’s model / Rutherford’s model).
(c) A classical atom based on _____ is doomed to collapse. (Thomson’s model / Rutherford’s model)
(d) An atom has a nearly continuous mass distribution in a _____ but has a highly non-uniform mass distribution in _____ (Thomson’s model / Rutherford’s model).
(e) The positively charged part of the atom possesses most of the mass in _____ (Rutherford’s model / both the models).
Solution:
(a) not different from
(b) Thomson’s model, Rutherford’s model
(c) Rutherford’s model
(d) Thomson’s model, Rutherford’s model
(e) both the models

Question 2. ( Atoms Notes )
Suppose you are given a chance to repeat the alpha-particle scattering experiment using a thin sheet of solid hydrogen in place of the gold foil. (Hydrogen is a solid at temperatures below 14 K). What results do you expect?
Solution:
The nucleus of a hydrogen atom is a proton. The mass of a proton is 1.67 × 10^-27 kg, whereas the mass of an incident a-particle is 6.64 × 10^-27 kg. Because the incident a-particles are more massive than the target nuclei (protons), the a-particle won’t bounce back even in a head on collision. It is similar to a football colliding with a tennis ball at rest. Thus, there would be no appreciable scattering.

Question 3. ( Atoms Notes )
What is the shortest wavelength present in the Paschen series of spectral lines?
Solution:
For shortest wavelength of Paschen series, n₁ = 3, n₂ = ∞

Question 4. ( Atoms Notes )
A difference of 2.3 eV separates two energy levels in an atom. What is the frequency of radiation emitted when the atom makes a transition from the upper level to the lower level?
Solution:

Question 5. ( Atoms Notes )
The ground state energy of hydrogen atom is -13.6 eV. What are the kinetic and potential energies of the electron in this state?
Solution:

Question 6. ( Atoms Notes )
A hydrogen atom initially in the ground level absorbs a photon, which excites it to the n = 4 level. Determine the wavelength and frequency of photon.
Solution:
Energy of an electron in the nth orbit of

Question 7.
(a) Using the Bohr’s model, calculate the speed of the electron in a hydrogen atom in the n = 1, 2 and 3 levels,
(b) Calculate the orbital period in each of these levels.
Solution:
(a) Speed of the electron in Bohr’s nth orbit is

(b) Orbital period of electron in Bohr’s first orbit is


Question 8.
The radius of the innermost electron orbit of a hydrogen atom is 5.3 × 10^-11 m. What are the radii of the n = 2andn = 3 orbits?
Solution:
Radius of innermost electron

Question 9.
A 12.5 eV electron beam is used to bombard gaseous hydrogen at room temperature. What series of wavelengths will be emitted?
Solution:
In ground state, energy of gaseous hydrogen at room temperature=-13.6 eV. When it is bombarded with 12.5 eV electron beam, the energy becomes – 13.6 + 12.5 = – 1.1 eV. The electron would jump from n = 1 to n = 3, 13 6 where E₃ =  = -1.5 eV. On de-excitation the electron may jump from n = 3 to n = 2 giving rise to Balmer series. It may also jump from n = 3 to n = 1, giving rise to Lyman series.

Question 10.
ln accordance with the Bohr’s model, find the quantum number that characterises the earth’s revolution around the sun in an orbit of radius 1.5 × 10¹¹ m with orbital speed 3 × 10⁴ m s^-1. (Mass of earth = 6.0 × 102²⁴kg)
Solution:
According to Bohr’s quantization condition of angular momentum, Angular momentum of the earth around the sun,

Question 11.
Answer the following questions, which helpyou understand the difference between Thomson’s model and Rutherford’s model better.
(a) Is the average angle of deflection of a-particles by a thin gold foil predicted by Thomson’s model much less, about the same, or much greater than that predicted by Rutherford’s model?
(b) Is the probability of backward scattering (i.e., scattering of a-particles at angles greater than 90°) predicted by Thomson’s model much less, about the same, or much greater than that predicted by Rutherford’s model?
(c) Keeping other factors fixed, it is found experimentally that for small thickness t, the number of a-particles scattered at moderate angles is proportional to What clue does this linear dependence on f provide?
(d) In which model is it completely wrong to ignore multiple scattering for the calculation of average angle of scattering of a-particles by a thin foil?
Solution:
(a) Nearly the same. This is because we are considering the average angle of deflection.
(b) Much less, because there is no such massive core (nucleus) in Thomson’s model as in Rutherford’s model.
(c) This suggests that scattering is mainly due to a single collision, because the chance of a single collision increases linearly with the number of the target atoms, and hence linearly with the thickness of the foil.
(d) In Thomson model, positive charge is distributed uniformly in the atom. So single collision causes very little deflection. The observed average scattering angle can be explained only by considering multiple scattering. Hence, it is wrong to ignore multiple scattering in Thomson’s model.

Question 12.
The gravitational attraction between electron and proton in a hydrogen atom is weaker than the coulomb attraction by a factor of about 10^-40. An alternative way of looking at this fact is to estimate the radius of the first Bohr orbit of a hydrogen atom if the electron and proton were bound by gravitational attraction. You will find the answer interesting.
Solution:
The radius of the first orbit in Bohr’s model is calculated by considering the electrostatic force between electrons and proton in nucleus.

This radius is much greater than the estimated size of the whole universe.

Question 13.
Obtain an expression for the frequency of radiation emitted when a hydrogen atom de-excites from level n to level (n – 1). For large n, show that this frequency equals the classical frequency of revolution of the electron in the orbit.
Solution:
Let us first find the frequency of revolution of electron in the orbit classically. In Bohr’s model velocity of electron in nth

Now, let us find the frequency of radiation emitted when a hydrogen atom de-excites from level h to level (n – 1).

Equation (i) and (ii) are equal, hence for large value of n, the classical frequency of revolution of electron in nth orbit is same as frequency of radiation when electron de-excite from level n to(n – l).

Question 14. ( Atoms Notes )
Classically, an electron can be in any orbit around the nucleus of an atom. Then what determines the typical atomic size? Why is an atom not, say, thousand times bigger than its typical size? The question had greatly puzzled Bohr before he arrived at his famous model of the atom that you have learnt in the text. To simulate what he might well have done before his discovery, let us play as follows with the basic constants of nature and see if we can get a quantity with the dimensions of length that is roughly equal to the known size of an atom 10 10 m)
(a) Construct a quantity with the dimensions of length from the fundamental constants e, m_e, and c. Determine its numerical value.
(b) You will find that the length obtained in (a) is many orders of magnitude smaller than the atomic dimensions. Further, it involves c. But energies of atoms are mostly in non-relativistic domain where c is not expected to play any role. This is what may have suggested Bohrto discard c and look for ‘something else’ to get the right atomic size. Now, the Planck’s constant h had already made its appearance elsewhere. Bohr’s great insight lay in recognising that h, m_e, and e will yield the right atomic size. Construct a quantity with the dimension of length from h, m_e, and e and confirm that its numerical value has indeed the correct order of magnitude.
Solution:

(b) A quantity with the dimension of length from h, m_e and e

The length is of the order of atomic size (10^-10m)

Question 15. ( Atoms Notes )
The total energy of an electron in the first excited state of the hydrogen atom is about – 3.4 eV.
(a) What is the kinetic energy of the electron in this state?
(b) What is the potential energy of the electron in this state?
(c) Which of the answers above would change if the choice of the zero of potential energy is changed?
Solution:
Kinetic energy of an electron in an orbit,

(a) Kinetic energy of electron in this state E = -K
(b) Potential energy E = U/2, U = 2E = 2 (-3.4) = -6.8 eV
(c) If thg zero of the potential energy is chosen differently, the kinetic energy remain the same. Although potential energy and hence total energy changes.

Question 16. ( Atoms Notes )
If Bohr’s quantisation postulate (angular momentum = nh/2n) is a basic law of nature, it should be equally valid for the case of planetary motion also. Why then do we never speak of quantisation of orbits of planets around the sun?
Solution:
Angular momentum mvr = n  associated with planetary motion are incomparably large relative to h. For example angular momentum of earth in its orbital motion is of the order of 10⁷⁰ .
For such large value of n, the difference in successive energies and angular momenta of the quantised levels of the Bohr model are so small that one can predict the energy level continuous.

Question 17. ( Atoms Notes )
Obtain the first Bohr’s radius and the ground state energy of a muonic hydrogen atom [/.e., an atom in which a negatively charged muon (μ) of mass about 207 me orbits around a proton].
Solution:
In Bohr’s model, the radius of nth orbit,

In the given muonic hydrogen atom, a negatively charged muon (μ^–) of mass 207 me revolve around a proton. Therefore radius of electron and muon can be written as

Related Links

Class 9th

Class 10th

Class 11th

Class 12th