[pdf id=’20901′]
4.1. Two charged particles traverse identical helical paths in an opposite sense in a uniform magnetic field

a) they have equal z-components of momenta
b) they must have equal charges
c) they necessarily represent a particle-antiparticle pair
d) the charge to mass ratio satisfy: (e/m)1 + (e/m)2 = 0
Answer:
d) the charge to mass ratio satisfy: (e/m)1 + (e/m)2 = 0
4.2. Biot-Savart law indicates that the moving electrons produce a magnetic field B such that
a) B ┴ v
b) B ‖ v
c) it obeys inverse cube law
d) it is along the line joining the electrons and point of observation
Answer:
a) B ┴ v
4.3. A current circular loop of radius R is placed in the x-y plane with centre at the origin. Half of the lop with x > 0 is now bent so that it now lies in the y-z plane.
a) the magnitude of magnetic moment now diminishes
b) the magnetic moment does not change
c) the magnitude of B at (0,0,z),z >> R increases
d) the magnitude of B at (0,0,z),z >> R is unchanged
Answer:
a) the magnitude of magnetic moment now diminishes
4.4. An electron is projected with uniform velocity along the axis of a current-carrying long solenoid. Which of the following is true?
a) the electron will be accelerated along the axis
b) the electron path will be circular about the axis
c) the electron will experience a force at 45o to the axis and hence execute a helical path
d) the electron will continue to move with uniform velocity along the axis of the solenoid
Answer:
d) the electron will continue to move with uniform velocity along the axis of the solenoid
4.5. In a cyclotron, a charged particle
a) undergoes acceleration all the time
b) speeds up between the dees because of the magnetic field
c) speeds up in a dee
d) slows down within a dee and speeds up between dees
Answer:
a) undergoes acceleration all the time
4.6. A circular current loop of magnetic moment M is in an arbitrary orientation in an external magnetic field B. The work done to rotate the loop by 30o about an axis perpendicular to its plane is
a) MB
b) √3 MB/2
c) MB/2
d) zero
Answer:
d) zero
Multiple Choice Questions II
4.7. The gyro-magnetic ratio of an electron in an H-atom, according to Bohr model is
a) independent of which orbit it is in
b) negative
c) positive
d) increases with the quantum number n
Answer:
a) independent of which orbit it is in
b) negative
4.8. Consider a wire carrying a steady current, I placed un a uniform magnetic field B perpendicular to its length. Consider the charges inside the wire. It is known that magnetic forces do not work. This implies that
a) motion of charges inside the conductor is unaffected by B since they do not absorb energy
b) some charges inside the wire move to the surface as a result of B
c) if the wire moves under the influence of B, no work is done by the force
d) if the wire moves under the influence of B, no work is done by the magnetic force on the ions, assumed fixed within the wire
Answer:
b) some charges inside the wire move to the surface as a result of B
d) if the wire moves under the influence of B, no work is done by the magnetic force on the ions, assumed fixed within the wire
4.9. Two identical current-carrying coaxial loops, carry current I in an opposite sense. A simple amperian loop passes through both of them once. Calling the loop as C,
a)
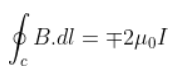
b) the value of
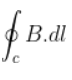
is independent of sense of C
c) there may be a point on C where B and dl are perpendicular
d) B vanishes everywhere on C
Answer:
b) the value of
is independent of sense of C
c) there may be a point on C where B and dl are perpendicular
4.10. A cubical region of space is filled with some uniform electric and magnetic fields. An electron enters the cube across one of its faces with velocity v and a positron enters via opposite face with velocity –v. At this instant,
a) the electric forces on both the particles cause identical acceleration
b) the magnetic forces on both the particles cause equal accelerations
c) both particles gain or lose energy at the same rate
d) the motion of the centre of mass (CM) is determined by B alone
Answer:
b) the magnetic forces on both the particles cause equal accelerations
c) both particles gain or lose energy at the same rate
d) the motion of the centre of mass (CM) is determined by B alone
4.11. A charged particle would continue to move with a constant velocity in a region wherein,
a) E = 0, B ≠ 0
b) E ≠ 0, B ≠ 0
c) E ≠ 0, B = 0
d) E = 0, B = 0
Answer:
a) E = 0, B ≠ 0
b) E ≠ 0, B ≠ 0
d) E = 0, B = 0
Very Short Answers
4.12. Verify that the cyclotron frequency ꞷ = eB/m has the correct dimensions of [T]-1.
Answer:
The path traced by the particle in a cyclotron is a circular path in which magnetic force acts as a centripetal force
mv2/R = evB
eB/m = v/R = ꞷ
B = F/ev = [MLT-2]/[AT][LT-1] = [MA-1T-2] [ꞷ] = [eB/m] = [v/R] = [T]-1
4.13. Show that a force that does no work must be a velocity dependent force.
Answer:

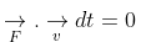
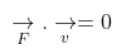
4.14. The magnetic force depends on v which depends on the inertial frame of reference. Does then the magnetic force differ from inertial frame to frame? Is it reasonable that the net acceleration has a different value in different frames of reference?
Answer:
The net acceleration can have a different value in different frames of reference as velocity depends on frame of reference.
4.15. Describe the motion of a charged particle in a cyclotron if the frequency of the radio frequency (rf) field were doubled.
Answer:
When the frequency of the radio frequency field was doubled, the time period of the radio frequency is halved which results in half revolution of the charges.
4.16. Two long wires carrying current I1 and I2 are arranged as shown in the figure. The one carrying I1 is along is the x-axis. The other carrying current I2 is along a line parallel to the y-axis given by x = 0 and z = d. Find the force exerted at O2 because of the wire along the x-axis.

Answer:
The magnetic field B on a current-carrying conductor is given as F = I(L×B) = ILB sinθ
O2 and I1 are parallel to the y-axis and are in the direction of –Y
I2 is parallel to the y-axis and is along Y-axis therefore, the angle between I2 and B1 is zero. The magnetic force F2 is given as F2 = B1I2L1 sin 0o = 0
Therefore, the force on O2 has current I1 zero.
Short Answers
4.17. A current-carrying loop consists of 3 identical quarter circles of radius R, lying in the positive quadrants of the x-y, y-z, and z-x planes with their centres at the origin, joined together. Find the direction and magnitude of B at the origin.
Answer:
The vector sum of the magnetic field at the origin due to the quarter is given as

4.18. A charged particle of charge e and mass m is moving in an electric field E and magnetic field B. Construct dimensionless quantities and quantities of dimension [T]-1.
Answer:
mv2/R = evB
eB/m = v/R = ꞷ
B = F/ev = [MA-1T-2] [ꞷ] = [eB/m]=[v/R] = [T-1]
4.19. An electron enters with a velocity v = v0i into a cubical region in which there are uniform electric and magnetic fields. The orbit of the electron is found to spiral down inside the cube in the plane parallel to the x-y plane. Suggest a configuration of fields E and B that can lead to it.
Answer:
The configuration of the fields E and B are the spiral path.4.20. Do magnetic forces obey Newton’s third law. Verify for two current elements.
4.20. Do magnetic forces obey Newton’s third law. Verify for two current elements
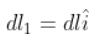
located at the origin and
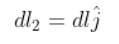
located at (0,R,0). Both carry current I.
Answer:
The magnetic forces do not obey Newton’s third law if there is no current flowing in the conductor which is placed parallel to each other.
4.21. A multirange voltmeter can be constructed by using a galvanometer circuit as shown in the figure. We want to construct a voltmeter that can measure 2V, 20V, and 200V using galvanometer of resistance 10Ω and that produces maximum deflection for a current of 1 mA. Find R1, R2, and R3 that have to be used.
Answer:
iG(G+R1) = 2 for 2V range
iG(G+R1+R2) = 20 for 20V range
iG(G+R1+R2+R3) = 200 for 200V range
Solving the above, we get
R1 = 1990 Ω
R2 = 18kΩ
R3 = 180 kΩ
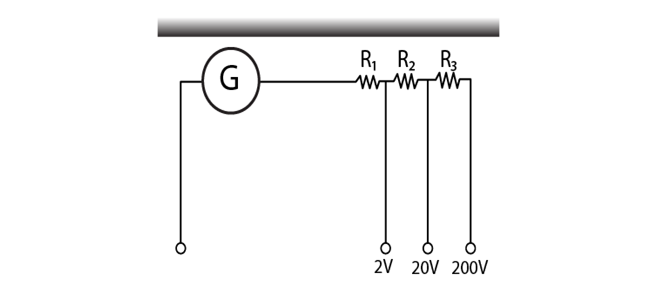
4.22. A long straight wire carrying a current of 25 A rests on a table as shown in the figure. Another wire PQ of length 1 m, mass 2.5 g carries the same current but in the opposite direction. The wire PQ is free to slide up and down. To what height will PQ rise?
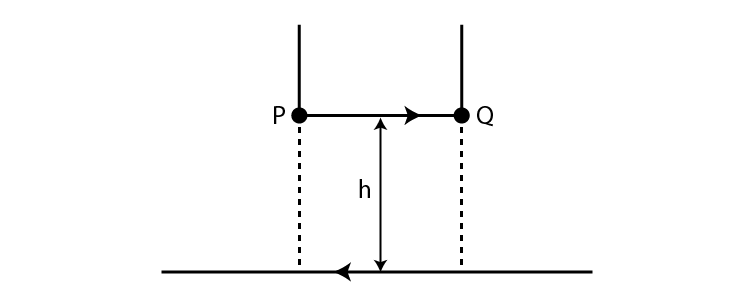
Answer:
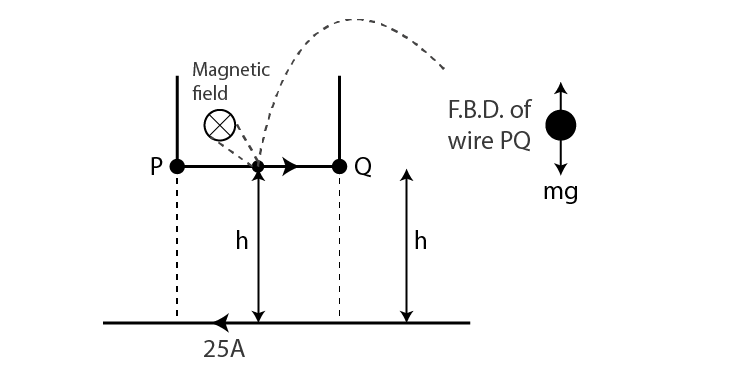
The magnetic field produced by a long straight current-carrying wire is given as
B = μ0I/2πh
Magnetic force on the small conductor is F = BIl sin θ = BIl
F = mg = μ0I2l/2πh
h = 0.51 cm
Long Answers
4.23. A 100 turn rectangular coil ABCD is hung from one arm of a balance. A mass 500 g is added to the other arm to balance the weight of the coil. A current 4.9 A passes through the coil and a constant magnetic field of 0.2 T acting inward is switched on such that only arm CD of length 1 cm lies in the field. How much additional mass ‘m’ must be added to regain the balance?
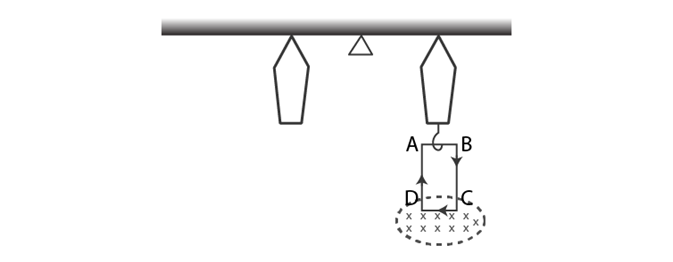
Answer:
When t = 0, the external magnetic field is off.
Mgl = Wcoil l
0.5 gl = Wcoil l
Wcoil = 0.5 9.8 N
Let m be the mass which is added to regain the balance
When the magnetic field is switched is on,
Mgl + mgl = (ILC)l
m = 1 g
4.24. A rectangular conducting loop consists of two wires on two opposite sides of length l joined together by rods of length d. The wires are each of the same material but with cross-sections differing by a factor of 2. The thicker wire has a resistance R and the rods are of low resistance, which in turn are connected to a constant voltage source Vo. The loop is placed in uniform a magnetic field B at 45oto its plane. Find τ, the torque exerted by the magnetic field on the loop about an axis through the centres of rods.
Answer:

Force and torque on the first wire is given as
F1 = i1l B sin 90o = V0/2R lB
τ1= d/2√2
F1 = V0ldB/2√2 R
τ = 1/4√2 V0AB/R4.25. An electron and a positron are released from (0, 0, 0) and (0, 0, 1.5R) respectively, in a uniform magnetic field
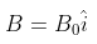
each with an equal momentum of magnitude p = eBR. Under what conditions on the direction of momentum will the orbits be non-intersecting circles?
Answer:
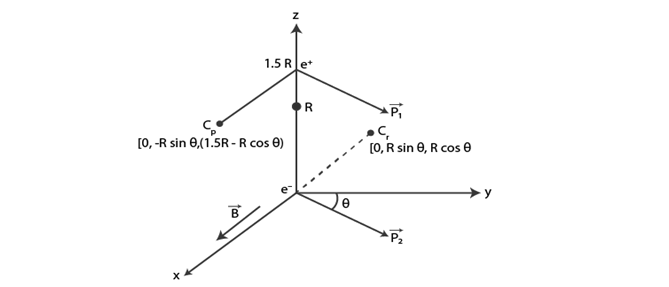
When the centres are greater than 2R, then the circular orbits of electron and positron shall not overlap.
Let d be the distance between Cp and Ce
Then d2 = 4R2 + 9/4R2 – 6R2 cosθ
As d is greater than 2R,
9/4 > 6 cos θ or cos θ < 3/8
4.26. A uniform conducting wire of length 12a and resistance R is wound up as a current-carrying coil in the shape of i) an equilateral triangle of side a; ii) a square if sides a and iii) a regular hexagon of sides a. The coil is connected to a voltage source V0. Find the magnetic moment of the coils in each case.
Answer:
a) An equilateral triangle with side a
No.of loops = 4
Area of the triangle A = √3/4 a2
Magnetic moment, m = Ia2√3
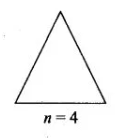
b) For a square with sides a
Area, A = a2
No.of loops = 3
Magnetic moment, m = 3Ia2

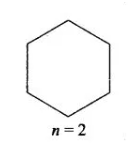
c) For a regular hexagon with sides a
Area, A = 6√3/4 a2
No.of loops = 2
Magnetic moment, m = 3√3a2I
Some of the concepts that are introduced in this chapter are:
- Ampere’s circuital law
- The Solenoid and the Toroid
- Moving Coil Galvanometer
- Magnetic Force
- Magnetic Field
1 Mark Questions ( Moving Charge And Magnetism )
1. State two properties of the material of the wire used for suspension of the coil in a moving coil galvanometer?
Ans. (a) Non-Brittle conductor
(b) Restoring Torque per unit Twist should be small.
2. What will be the path of a charged particle moving along the direction of a uniform magnetic field?
Ans. The path of a charged particle will be a straight line path as no force acts on the particle.
3. Two wires of equal lengths are bent in the form of two loops. One of the loop is square shaped whereas the other loop is circular. These are suspended in a uniform magnetic field and the same current is passed through them. Which loop will experience greater torque? Give reasons?
Ans. since
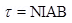
Since Area of – circular loops is more Than of a square loop
Torque experienced by a circular loop is greater.
4. A cyclotron is not suitable to accelerate electron. Why?
Ans. A cyclotron is not suitable to accelerate electron because its mass is less due to which they gain speed and step out of the dee immediately.
2 Marks Questions ( Moving Charge And Magnetism )
1. A steady current flows in the network shown in the figure. What will be the magnetic field at the centre of the network?
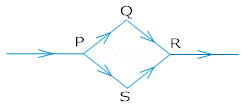
Ans. Zero, because magnetic field at the centre of the loop is just equal and opposite i.e. magnetic field due 1- PQR is equal and opposite to that of PSR.
2. An – particle and a proton are moving in the plane of paper in a region where there is uniform magnetic field B directed normal to the plane of paper. If two particles have equal linear momenta, what will be the ratio of the radii of their trajectories in the field?

3. Give one difference each between diamagnetic and ferromagnetic substances. Give one example of each?
Ans. Diamagnetic substances are weakly repelled by a magnet eg. Gold.
Ferromagnetic materials are strongly attracted by a magnet eg. Iron.
4. Write the expression for the force acting on a charged particle of charge q moving with velocity is in the presence of magnetic field B. Show that in the presence of this force.
(a) The K.E. of the particle does not change.
(b) Its instantaneous power is zero.
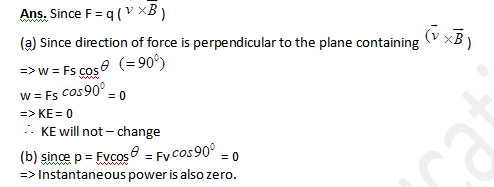
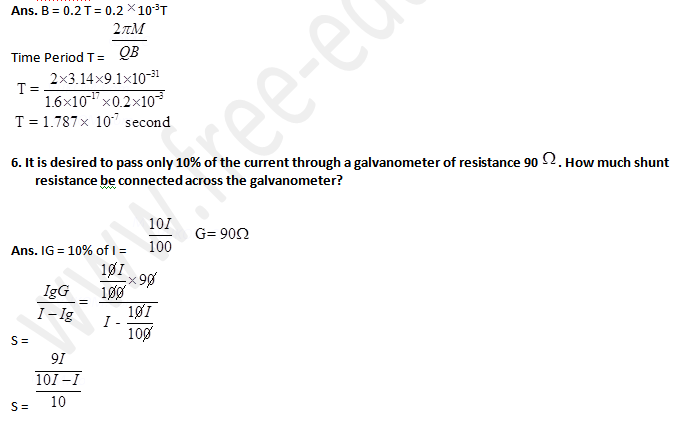
5. An electron of kinetic energy 25KeV moves perpendicular to the direction of a uniform magnetic field of 0.2 millitesla calculate the time period of rotation of the electron in the magnetic field?
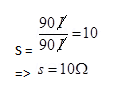
3 Marks Questions ( Moving Charge And Magnetism )
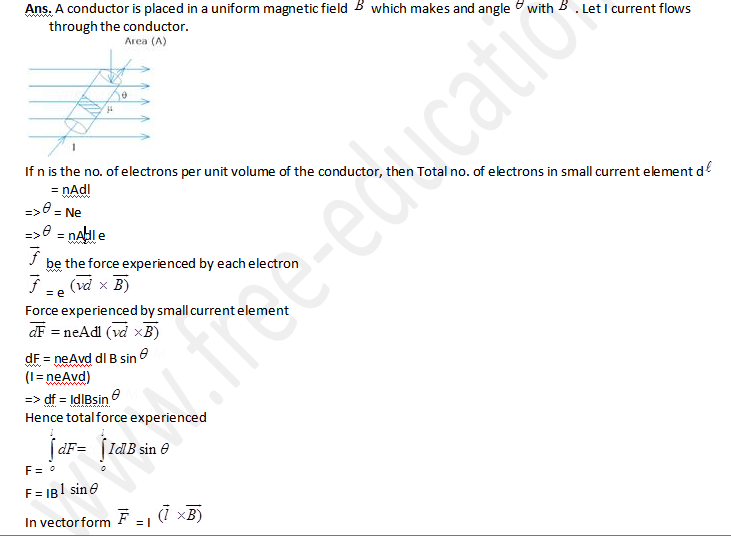
1. Derive an expression for the force acting on a current carrying conductor placed in a uniform magnetic field Name the rule which gives the direction of the force. Write the condition for which this force will have (1) maximum (2) minimum value?
Ans. A conductor is placed in a uniform magnetic field which makes and angle with . Let I current flows through the conductor.



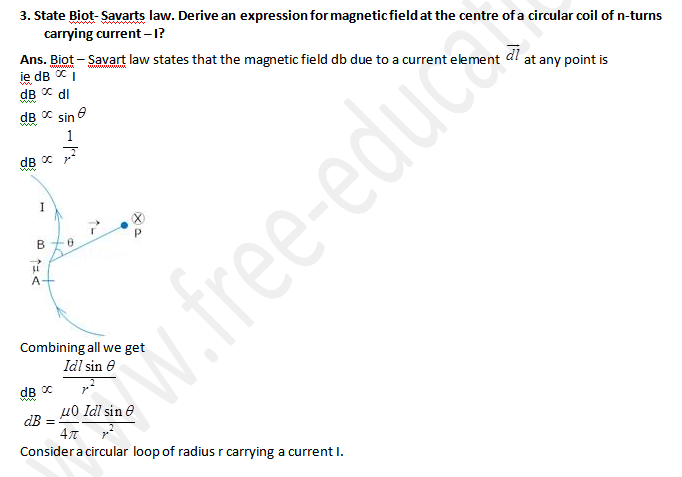
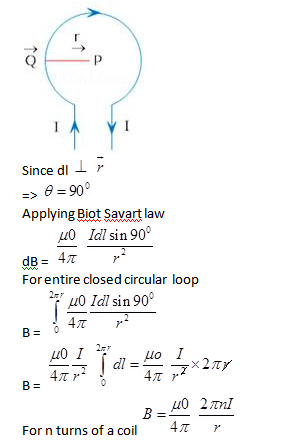

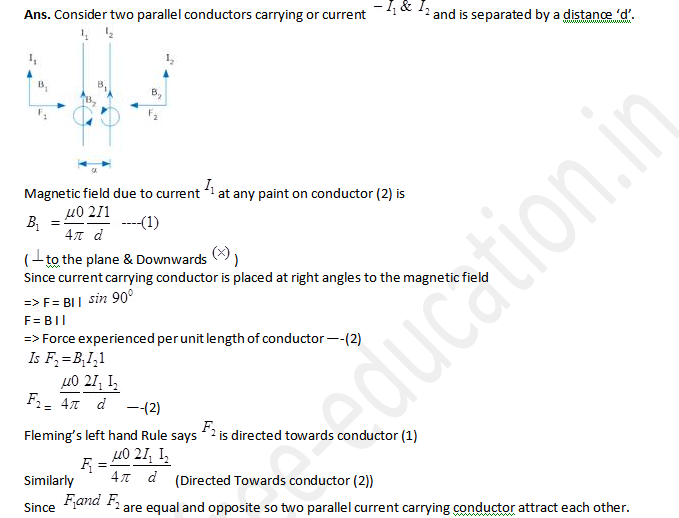

5 Marks Questions
1. (a) What is cyclotron? Explain its working principle?
(b) A cyclotron’s oscillator frequency is 10MHz what should be the operating magnetic field for accelerating protons? If radius of its dees is 20cm, what is the K.E. of the proton beam produced by the accelerator? (, ,)?
Ans. (a) It is a device used to accelerate charged particles like protons, deuterons, – particle etc.
It is based on the principle that a charged particle can be accelerated to very high energies by making it pass through a moderate electric field a number of times and applying a strong magnetic field at the same time.
(b) v = 10MHz = 10106 Hz
r = 20cm =
KE =
Since
2. (a) Draw a labelled diagram of a moving coil galvanometer. Prove that in a radial magnetic field, the deflection of the coil is directly proportional to the current flowing in the coil.
(b) A galvanometer can be converted into a voltmeter to measure upto
(i) V volt by connecting a resistance series with the coil
(ii) volt by connecting a resistance in series with coil Find R in terms of required to convert – it into a voltmeter that can read upto ‘2v’ volt.
Ans. (a) When a current I is passed through a coil two equal and opposite forces acts on the arms of a coil to form a couple which exerts a Torque on the coil.
=>
If =
is the angle made by the normal to the plane of coil with B
= NIAB —-(1)
This is called as deflecting torque
As the coil deflected the spring is twisted and a restoring torque per unit twist then the restoring torque for the deflecting & is given by
= k —-(2)
In equilibrium
Deflecting Torgue=Restoring Torgue
NIAB = K
I =
I = G where G = (galvanometer constant)
=>
Thus deflection of the coil is directly proportional to the current flowing in the coil.
(b) We know Ig =
=> Ig = —–(1)
And Ig =
Equating (1) & (2)
For conversion Ig =
=> Ig
3. Two moving coil meters, M1 and M2 have the following particulars:
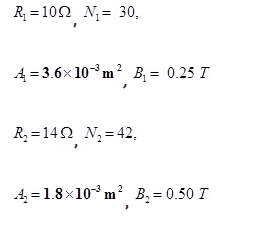
(The spring constants are identical for the two meters).
Determine the ratio of (a) current sensitivity and (b) voltage sensitivity of
Ans. For moving coil meter M1:
Resistance,
Number of turns,
Area of cross-section,
Magnetic field strength,
Spring constant
For moving coil meter M2:
Resistance,
Number of turns,
Area of cross-section,
Magnetic field strength,
Spring constant,
(a) Current sensitivity of is given as:
And, current sensitivity of is given as:
Ratio
Hence, the ratio of current sensitivity of is 1.4.
(b) Voltage sensitivity for is given as:
And, voltage sensitivity for is given as:
4. In a chamber, a uniform magnetic field of 6.5 G is maintained. An electron is shot into the field with a speed of normal to the field. Explain why the path of the electron is a circle. Determine the radius of the circular orbit. (e =, me=)
Ans.Magnetic field strength, B = 6.5 G =
Speed of the electron, v =
Charge on the electron, e =
Mass of the electron,
Angle between the shot electron and magnetic field,
Magnetic force exerted on the electron in the magnetic field is given as:
F = evB
This force provides centripetal force to the moving electron. Hence, the electron starts moving in a circular path of radius r.
Hence, centripetal force exerted on the electron,
In equilibrium, the centripetal force exerted on the electron is equal to the magnetic force i.e.,
= 4.2 cm
Hence, the radius of the circular orbit of the electron is 4.2 cm.
5. In Exercise 4.11 obtain the frequency of revolution of the electron in its circular orbit. Does the answer depend on the speed of the electron? Explain.
Ans. Magnetic field strength,
Charge of the electron,
Mass of the electron,
Velocity of the electron,
Radius of the orbit, r = 4.2 cm = 0.042 m
Frequency of revolution of the electron = v
Angular frequency of the electron = = 2nv
Velocity of the electron is related to the angular frequency as:
v = r
In the circular orbit, the magnetic force on the electron is balanced by the centripetal force. Hence, we can write:
This expression for frequency is independent of the speed of the electron.
On substituting the known values in this expression, we get the frequency as:
Hence, the frequency of the electron is around 18 MHz and is independent of the speed of the electron.
6. Two concentric circular coils X and Y of radii 16 cm and 10 cm, respectively, lie in the same vertical plane containing the north to south direction. Coil X has 20 turns and carries a current of 16 A; coil Y has 25 turns and carries a current of 18 A. The sense of the current in X is anticlockwise, and clockwise in Y, for an observer looking at the coils facing west. Give the magnitude and direction of the net magnetic field due to the coils at their centre.
Ans. Radius of coil X, = 0.16 m
Radius of coil Y, = 0.1 m
Number of turns of on coil X,
Number of turns of on coil Y,
Current in coil X,
Current in coil Y,
Magnetic field due to coil X at their centre is given by the relation,
Where,
= Permeability of free space =
(towards East)
Magnetic field due to coil Y at their centre is given by the relation,
(towards East)
Hence, net magnetic field can be obtained as:
7. For a circular coil of radius R and N turns carrying current I, the magnitude of the magnetic field at a point on its axis at a distance x from its centre is given by,
(a) Show that this reduces to the familiar result for field at the centre of the coil.
(b) Consider two parallel co-axial circular coils of equal radius R, and number of turns N, carrying equal currents in the same direction, and separated by a distance R. Show that the field on the axis around the mid-point between the coils is uniform over a distance that is small as compared to R, and is given by, , approximately. [Such an arrangement to produce a nearly uniform magnetic field over a small region is known as Helmholtz coils.]
Ans. Radius of circular coil = R
Number of turns on the coil = N
Current in the coil = I
Magnetic field at a point on its axis at distance x is given by the relation,
Where,
= Permeability of free space
(a) If the magnetic field at the centre of the coil is considered, then x = 0.
This is the familiar result for magnetic field at the centre of the coil.
(b) Radii of two parallel co-axial circular coils = R
Number of turns on each coil = N
Current in both coils = I
Distance between both the coils = R
Let us consider point Q at distance d from the centre.
Then, one coil is at a distance of from point Q.
Magnetic field at point Q is given as:
Also, the other coil is at a distance of from point Q.
Magnetic field due to this coil is given as:
Total magnetic field,
For d << R, neglecting the factor , we get:
Hence, it is proved that the field on the axis around the mid-point between the coils is uniform.
8. An electron emitted by a heated cathode and accelerated through a potential difference of 2.0 kV, enters a region with uniform magnetic field of 0.15 T. Determine the trajectory of the electron if the field (a) is transverse to its initial velocity, (b) makes an angle of 30º with the initial velocity.
Ans. Magnetic field strength, B = 0.15 T
Charge on the electron,
Mass of the electron,
Potential difference, V = 2.0
Thus, kinetic energy of the electron =
……(1)
Where,
v = velocity of the electron
(a) Magnetic force on the electron provides the required centripetal force of the electron. Hence, the electron traces a circular path of radius r.
Magnetic force on the electron is given by the relation,
B ev
Centripetal force
……(2)
From equations (1) and (2), we get
= 1 mm
Hence, the electron has a circular trajectory of radius 1.0 mm normal to the magnetic field.
(b) When the field makes an angle with initial velocity, the initial velocity will be,
From equation (2), we can write the expression for new radius as:
= 0.5 mm
Hence, the electron has a helical trajectory of radius 0.5 mm along the magnetic field direction.
9. A magnetic field set up using Helmholtz coils (described in Exercise 4.16) is uniform in a small region and has a magnitude of 0.75 T. In the same region, a uniform electrostatic field is maintained in a direction normal to the common axis of the coils. A narrow beam of (single species) charged particles all accelerated through 15 kV enters this region in a direction perpendicular to both the axis of the coils and the electrostatic field. If the beam remains undeflected when the electrostatic field is, make a simple guess as to what the beam contains. Why is the answer not unique?
Ans. Magnetic field, B = 0.75 T
Accelerating voltage, V = 15
Electrostatic field, E =
Mass of the electron = m
Charge of the electron = e
Velocity of the electron = v
Kinetic energy of the electron = eV
…… (1)
Since the particle remains unelected by electric and magnetic fields, we can infer that the electric field is balancing the magnetic field.
……..(2)
Putting equation (2) in equation (1), we get
This value of specific charge e/m is equal to the value of deuteron or deuterium ions. This is not a unique answer. Other possible answers are, etc.
10. A uniform magnetic field of 1.5 T exists in a cylindrical region of radius10.0 cm, its direction parallel to the axis along east to west. A wire carrying current of 7.0 A in the north to south direction passes through this region. What is the magnitude and direction of the force on the wire if,
(a) the wire intersects the axis,
(b) the wire is turned from N-S to northeast-northwest direction,
(c) the wire in the N-S direction is lowered from the axis by a distance of 6.0 cm?
Ans.Magnetic field strength, B = 1.5 T
Radius of the cylindrical region, r = 10 cm = 0.1 m
Current in the wire passing through the cylindrical region, I = 7 A
(a) If the wire intersects the axis, then the length of the wire is the diameter of the cylindrical region.
Thus, l = 2r = 0.2 m
Angle between magnetic field and current,
Magnetic force acting on the wire is given by the relation,
F = BIl
=
= 2.1 N
Hence, a force of 2.1 N acts on the wire in a vertically downward direction.
(b) New length of the wire after turning it to the Northeast-Northwest direction can be given as:
Angle between magnetic field and current, θ = 45°
Force on the wire,
F =
= BIl
=2.1 N
Hence, a force of 2.1 N acts vertically downward on the wire. This is independent of angle because is fixed.
(c) The wire is lowered from the axis by distance, d = 6.0 cm
Suppose wire is passing perpendicularly to the axis of cylindrical magnetic field then lowering 6 cm means displacing the wire 6 cm from its initial position towards to end of cross sectional area.
= 8cm
Thus the length of wire in magnetic field will be 16 cm as AB= L =2x =16 cm
Now the force,
F = iLB as the wire will be perpendicular to the magnetic field.
The direction will be given by right hand curl rule or screw rule i.e. vertically downwards.
11. A uniform magnetic field of 3000 G is established along the positive z-direction. A rectangular loop of sides 10 cm and 5 cm carries a current of 12 A. What is the torque on the loop in the different cases shown in Fig. 4.28? What is the force on each case? Which case corresponds to stable equilibrium?
Ans.Magnetic field strength, B = 3000 G = = 0.3 T
Length of the rectangular loop, l = 10 cm
Width of the rectangular loop, b = 5 cm
Area of the loop,
= =
Current in the loop, I = 12 A
Now, taking the anti-clockwise direction of the current as positive and vise-versa:
(a) Torque,
From the given figure, it can be observed that A is normal to the y–z plane and B is directed along the z-axis.
The torque is along the negative y-direction. The force on the loop is zero because the angle between A and B is zero.
(b) This case is similar to case (a). Hence, the answer is the same as (a).
(c) Torque
From the given figure, it can be observed that A is normal to the x–z plane and B is directed along the z-axis.
The torque is along the negative x direction and the force is zero.
(d) Magnitude of torque is given as:
Torque is at an angle of with positive x direction. The force is zero.
(e) Torque
= 0
Hence, the torque is zero. The force is also zero.
(f) Torque
= 0
Hence, the torque is zero. The force is also zero.
In case (e), the direction of and is the same and the angle between them is zero. If displaced, they come back to an equilibrium. Hence, its equilibrium is stable.
Whereas, in case (f), the direction of and is opposite. The angle between them is 180°. If disturbed, it does not come back to its original position. Hence, its equilibrium is unstable.
12. A solenoid 60 cm long and of radius 4.0 cm has 3 layers of windings of 300 turns each. A 2.0 cm long wire of mass 2.5 g lies inside the solenoid (near its centre) normal to its axis; both the wire and the axis of the solenoid are in the horizontal plane. The wire is connected through two leads parallel to the axis of the solenoid to an external battery which supplies a current of 6.0 A in the wire. What value of current (with appropriate sense of circulation) in the windings of the solenoid can support the weight of the wire?
Ans.Length of the solenoid, L = 60 cm = 0.6 m
Radius of the solenoid, r = 4.0 cm = 0.04 m
It is given that there are 3 layers of windings of 300 turns each.
Total number of turns, = 900
Length of the wire, l = 2 cm = 0.02 m
Mass of the wire, m = 2.5 g =
Current flowing through the wire, i = 6 A
Acceleration due to gravity,
Magnetic field produced inside the solenoid,
Where,
= Permeability of free space =
I = Current flowing through the windings of the solenoid
Magnetic force is given by the relation, F = Bil
Also, the force on the wire is equal to the weight of the wire.
Hence, the current flowing through the solenoid is 108 A.