Close Packing in One Dimension
- The only way to arrange spheres in a one dimensional close packed structure is to arrange the spheres in a single row and touching each other.

- Each sphere is in contact with two of its surrounding atom.
- The number of nearest surrounding particles is called its coordination number. Therefore in one dimensional close packed arrangement, the coordination number is 2.
Close Packing in Two Dimensions
Two dimensional close packed structure can be generated by stacking (placing) the rows of close packed spheres.
The second row can be placed in contact with the first row in such a way that the spheres of second row are exactly above the first row.
- The spheres of the two rows are aligned horizontally as well as vertically.
- Let the first row be ‘A’ type row and the second row is also of ‘A’ type due to its similarity with the first one.
- Similarly, we may place more rows to obtain AAA type of arrangement.
- Each sphere is in contact with four of its surrounding atom. Thus, the two dimensional coordination numbers is 4.
- If the centers of these 4 immediate surrounding spheres are joined, a square is formed. Due to which this packing is known as square close packing in two dimensions.
- The second row can be placed above the first row in a staggered manner in such a way that the spheres fit in the depressions of the first row.
- Let the first row be ‘A’ type row, and the second row be ‘B’ type row.
- When the third row is placed adjacent to the second in staggered manner, its spheres are aligned with those of the first layer. Hence this layer is also of ‘A’ type.
- The spheres of similarly placed fourth row will be aligned with those of the second row (‘B’ type). Hence this arrangement is of ABAB type.
- This arrangement provides less free space and is more efficient than the square close packing.
- Each sphere in this arrangement is in contact with six of surrounding spheres and the two dimensional coordination numbers is 6.
- The centers of these six spheres are at the corners of a regular hexagon due to which this packing is also known as two dimensional hexagonal close packing.
- These are triangular in shape. In one row, the apex of the triangles is pointing upwards and in the next layer the apex of the triangles is pointing downwards.
Close Packing in Three Dimensions
All real structures are three dimensional structures that are obtained by stacking two dimensional layers one above the other in a square close-packed and hexagonal close-packed manner. It can be categorized into two types:
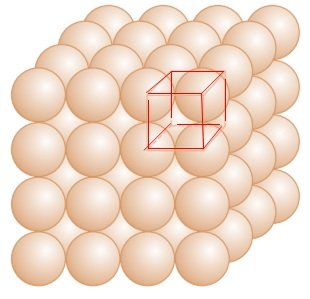
Three dimensional close packing from two dimensional square close-packed layers:
- The second layer is placed over the first layer in such a way that the spheres of the upper layer are exactly above those of the first layer.
- In this arrangement of spheres, both the layers are perfectly aligned horizontally as well as vertically.
- Let the first row be ‘A’ type row, and the second row be ‘B’ type row.
- Thus this lattice has AAA…. type pattern.
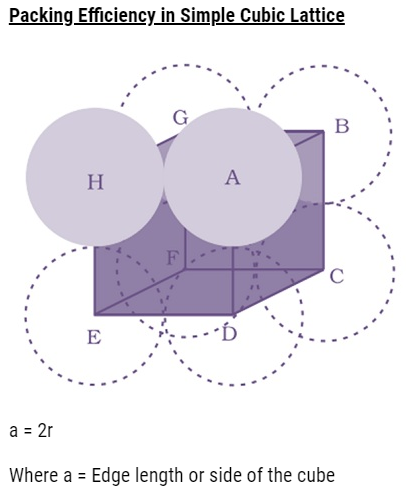
- The lattice therefore generated is the simple cubic lattice, and its unit cell is the primitive cubic unit cell.
Three dimensional close packing from two dimensional hexagonal close packed layers:
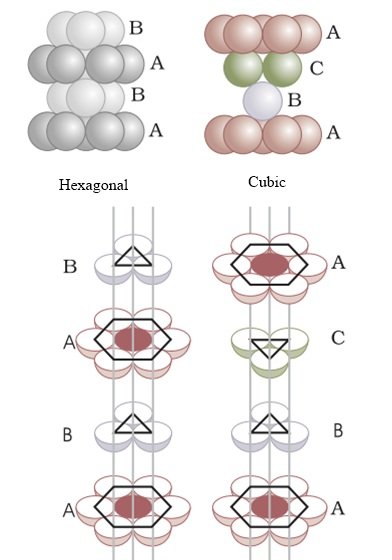
Three dimensional close packed structure can be generated by placing layers one over the other.
- Placing second layer over the first layer
Placing third layer over the second layer
Placing second layer over the first layer
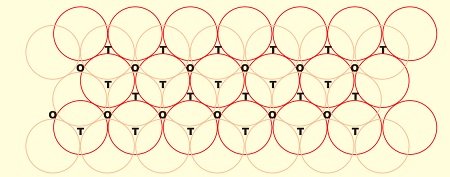
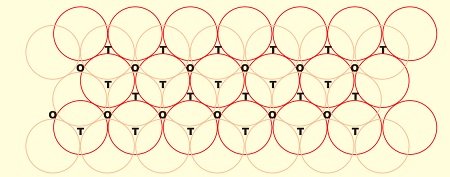
- Consider a two dimensional hexagonal close packed layer of type ‘A’ and place a similar layer above it in such a way that the spheres of the second layer lies in the depressions of the first layer.
- Let the second layer be of type ‘B’ due to its different alignment.
- All the triangular voids of the first layer are not covered by the spheres of the second layer which gives rise to a different arrangement.
- The area where a sphere of the second layer is above the void of the first layer (or vice versa) a tetrahedral void is formed.
- These voids are called tetrahedral voids because a joining of the centers of four spheres gives rise to tetrahedron.
- There are areas where the triangular voids in the second layer lies above the triangular voids of the first layer, and the triangular shapes of these do not overlap.
- One of them has the apex of the triangle pointing upwards and the other downwards.
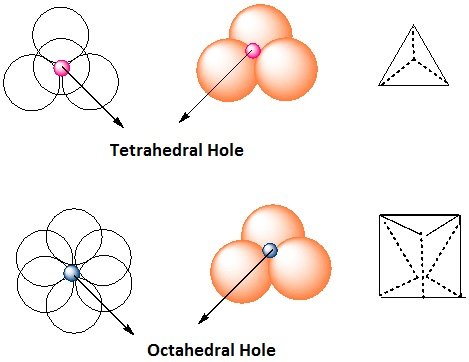
- Such voids are surrounded by six spheres and are called octahedral voids (O).
Let the number of close packed spheres be N, then:
The number of octahedral voids generated in the structure = N.
The number of tetrahedral voids generated in the structure = 2N.
Question : If the radius of the octachedral void is r and radius of the atoms in close packing is R, derive relation between r and R.
Answer: Given that
Radius of octahedral void in close packing = r
Radius of atom in close packing = R
Using Pythagoras theorem,
2R2 = (R+ r)2 + (R+ r)2
4R2 = 2(R +r)2
2R2 = (R+ r)2
R√2 = R +r
R√2 – R = r
r = R√2 – R
Putting the value of √2, we derive
r = R (1.414-1)
r = 0.414R
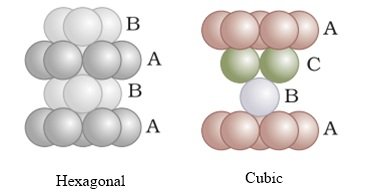
Placing third layer over the second layer : Covering Tetrahedral Voids
- Tetrahedral voids of the second layer can get covered by the spheres of the third layer.
- Here the spheres of the third layer get exactly aligned with the spheres of the first layer.
- Therefore it can be observed that the pattern of spheres is repeated in alternate layers.
- This pattern is often written as ABAB ……. pattern and the structure is called hexagonal close packed (hcp) structure found in many metals like magnesium and zinc.
Question : A compound forms hexagonal close-packed structure. What is the total number of voids in 0.5 mol of it? How many of these are tetrahedral voids?
Answer: Number of particles in 1 mol of compound = 6.022 X 1023
Number of particles in 0.5 mol = 0.5 X6.022 X 1023 = 3.011 X 1023
Number of octahedral voids = number of atoms or particles.
Number of tetrahedral voids = 2 X number of particles
Therefore number of octahedral in the given compound = 3.011 X 1023
Number of tetrahedral voids = 2 X 3.011 X 1023 = 6.022 X 1023
The total number of voids = 3.011 X 1023 + 6.022 X 1023
= 9.033 X 1023
Number of tetrahedral voids = 6.022 X 1023
Question. Atoms of element B form hcp lattice and those of the element A occupy 2/3rd of tetrahedral voids. What is the formula of the compound formed by the elements A and B?
Answer: Number of tetrahedral voids = 2 X number of particles of atom B
Number of atoms of A is equal to of the number of atoms of B.
Hence the ratio A:B = (4/3):1 or 4:3
Therefore, the formula of the compound is A4B3.
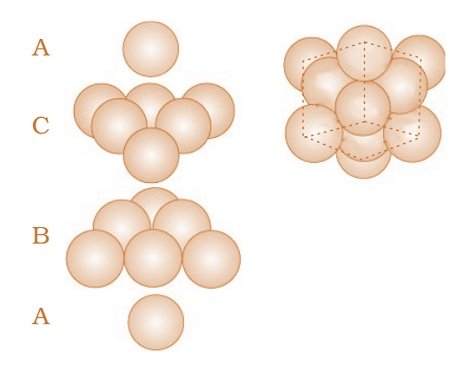
Placing third layer over the second layer : Covering Octahedral Voids
- The third layer may be placed above the second layer in a manner such that its spheres cover the octahedral voids.
- When placed in this manner, the spheres of the third layer are not aligned with
those of either the first or the second layer. This arrangement is called “C’ - Only when fourth layer is placed, its spheres are aligned with those of the first layer.
- This pattern of layers is often written as ABCABC……….. This structure is called cubic close packed (ccp) or face-centered cubic (fcc) structure.
- Metals such as copper and silver crystallize in this structure. Both these types of close packing are highly efficient and 74% space in the crystal is filled. In either of them, each sphere is in contact with twelve spheres. Thus, the coordination number is 12 in either of these two structures.
Question: A compound is formed by two elements M and N. The element N
forms ccp and atoms of M occupy 1/3rd of tetrahedral voids. What
is the formula of the compound?
Answer: The ccp lattice is made by the atoms of the element N.
Here, the Number of tetrahedral voids = 2 X number of particles of the elements N.
According to the question, the atoms of element M occupy = 1/3rd of the tetrahedral voids.
Therefore, the number of atoms of M is equal to
2 x 1/3 = 2/3rd of the number of atoms of N.
Therefore, the ratio M:N = 2/3 :1 = 2:3
Hence formula of the compound is M2N3.
Formula of a Compound and Number of Voids Filled
- The number of octahedral voids present in a lattice = The number of close packed particles.
- The number of tetrahedral voids produced is twice this number.
- In ionic solids, the bigger ions i.e. anions forms close packed structure and the smaller ions i.e. cations occupy the voids.
- If the latter ion is small then tetrahedral voids are occupied, if bigger, then octahedral voids are occupied.
- In a compound, the fraction of octahedral or tetrahedral voids that are occupied depends upon the chemical formula of the compound.
- Close packed structures have both tetrahedral and octahedral voids.
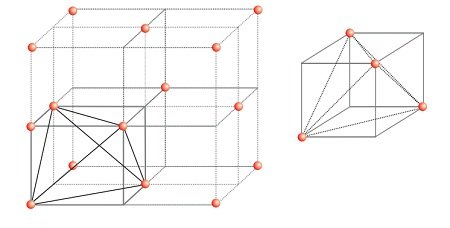
Locating Tetrahedral Voids
- Take a unit cell of ccp or fcc lattice divide into eight small cubes with each small cube having atoms at alternate corners.
- Thus, each small cube has 4 atoms that on joining to each other make a regular tetrahedron.
- Thus, there is one tetrahedral void in each small cube and eight tetrahedral voids in total.
- Each of the eight small cubes have one void in one unit cell of ccp
- ccp structure has 4 atoms per unit cell. Thus, the number of tetrahedral voids is twice the number of atoms.
Locating Octahedral Voids
- Take a unit cell of ccp or fcc.
- The body center of the cube, C is not occupied but surrounded by six atoms on face centers.
- Joining the face centers creates a octahedron.
- Thus, this unit cell has one octahedral void at the body centre of the cube.
- There is one octahedral void at the centre of each
of the 12 edges. - It is surrounded by six atoms, three belonging to the same unit cell and three belonging to
two adjacent unit cells. - Each edge of the cube and octahedral void are shared between four adjacent unit cells.
- Only ¼ of each void belongs to a particular unit cell
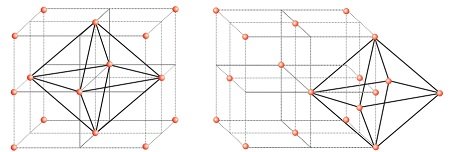
In cubic close packed structure octahedral void at the body-center of the cube = 1
12 octahedral voids positioned at each edge and shared between four unit cells =12 x 1/4 =3
Total number of octahedral voids = 4
In ccp structure, each unit cell has 4 atoms. Thus, the number
of octahedral voids is equal to this number.
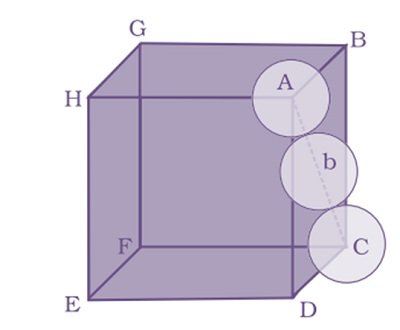
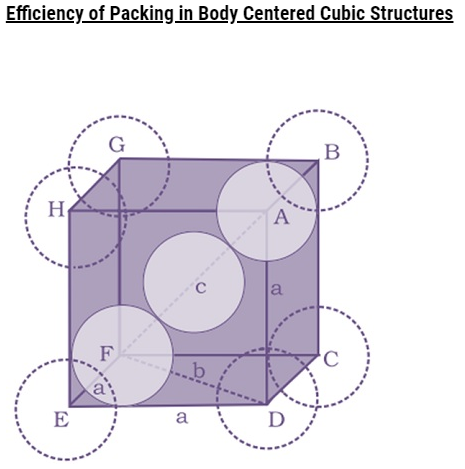
Packing Efficiency in hcp and ccp Structures
let the unit cell edge length be ‘a’ and face diagonal AC = b.
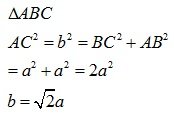


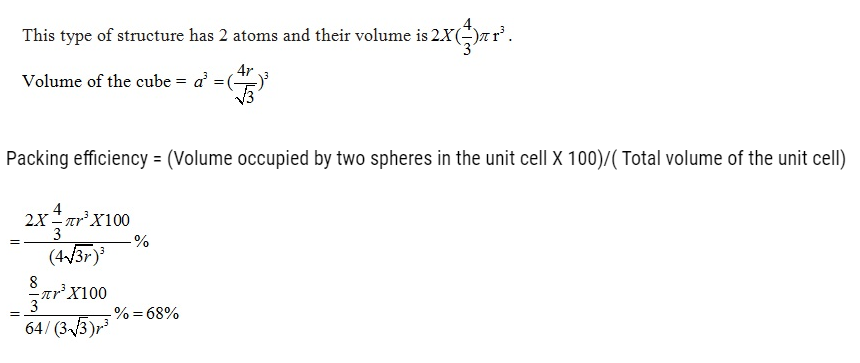



Mohd. Sharif Qualification: B.Tech (Mechanical Engineering) [Founder of Wisdom Academy] [Aim Foundation & Free-Education.In] [Engineer By Profession | Teacher By Choice] [Blogger, YouTube Creator]






