Introduction to Algebraic Expressions
Constant
Constant is a quantity which has a fixed value.
Terms of Expression
Parts of an expression which are formed separately first and then added are known as terms. They are added to form expressions.
Example: Terms 4x and 5 are added to form the expression (4x +5).
Coefficient of a term
The numerical factor of a term is called coefficient of the term.
Example: 10 is the coefficient of the term 10xy in the expression 10xy+4y.
Writing Number patterns and rules related to them
- If a natural number is denoted by n, its successor is (n + 1).
Example: Successor of n=10 is n+1 =11. - If a natural number is denoted by n, 2n is an even number and (2n+1) an odd number.
Example: If n=10, then 2n = 20 is an even number and 2n+1 = 21 is an odd number.
Writing Number patterns and rules related to them
- If a natural number is denoted by n, its successor is (n + 1).
Example: Successor of n=10 is n+1 =11. - If a natural number is denoted by n, 2n is an even number and (2n+1) an odd number.
Example: If n=10, then 2n = 20 is an even number and 2n+1 = 21 is an odd number.
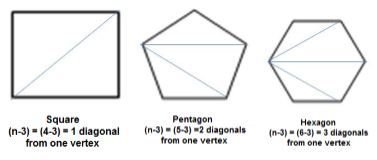
Writing Patterns in Geometry
- Algebraic expressions are used in writing patterns followed by geometrical figures.
Example: Number of diagonals we can draw from one vertex of a polygon of n sides is (n – 3).
Definition of Variables
- Any algebraic expression can have any number of variables and constants.
-
Variable
- A variable is a quantity that is prone to change with the context of the situation.
- a,x,p,… are used to denote variables.
-
-
-
Constant
-
- It is a quantity which has a fixed value.
- In the expression 5x+4, the variable here is x and the constant is 4.
- The value 5x and 4 are also called terms of expression.
- In the term 5x, 5 is called the coefficient of x. Coefficients are any numerical factor of a term.
Factors of a term
Factors of a term are quantities which can not be further factorised. A term is a product of its factors.
Example: The term –3xy is a product of the factors –3, x and y.
Formation of Algebraic Expressions
- Variables and numbers are used to construct terms.
- These terms along with a combination of operators constitute an algebraic expression.
- The algebraic expression has a value that depends on the values of the variables.
- For example, let 6p2−3p+5 be an algebraic expression with variable p
The value of the expression when p=2 is,
6(2)2 − 3(2) + 5
⇒ 6(4) − 6 + 5 = 23
The value of the expression when p=1 is,
6(1)2 − 3(1) + 5
⇒ 6 − 3 + 5 = 8
Like and Unlike Terms
Like terms
- Terms having same algebraic factors are like terms.
Example: 8xy and 3xy are like terms.
Unlike terms
- Terms having different algebraic factors are unlike terms.
Example: 7xy and −3x are unlike terms.
Monomial, Binomial, Trinomial and Polynomial Terms
Types of expressions based on the number of terms
Based on the number of terms present, algebraic expressions are classified as:
- Monomial: An expression with only one term.
Example: 7xy, −5m, etc. - Binomial: An expression which contains two, unlike terms.
Example: 5mn+4, x+y, etc - Trinomial: An expression which contains three terms.
Example: x+y+5, a+b+ab, etc.
Polynomials
- An expression with one or more terms.
Example: x+y, 3xy+6+y, etc.
Addition and Subtraction of Algebraic Equations
- Mathematical operations like addition and subtraction can be applied to algebraic terms.
- For adding or subtracting two or more algebraic expression, like terms of both the expressions are grouped together and unlike terms are retained as it is.
- Sum of two or more like terms is a like term with a numerical coefficient equal to the sum of the numerical coefficients of all like terms.
- Difference between two like terms is a like term with a numerical coefficient equal to the difference between the numerical coefficients of the two like terms.
- For example, 2y + 3x − 2x + 4y
⇒ x(3−2) + y(2+4)
⇒ x+6y - Summation of algebraic expressions can be done in two ways:
Consider the summation of the algebraic expressions 5a2+7a+2ab and 7a2+9a+11b - Horizontal method
5a2+7a+2ab+7a2+9a+11b
= (5+7)a2+(7+9)a+2ab+11b
= 12a2+16a+2ab+11b - Vertical method
5a2+7a+2ab
7a2+9a+11b
Addition and Subtraction of Algebraic Equations
- Mathematical operations like addition and subtraction can be applied to algebraic terms.
- For adding or subtracting two or more algebraic expression, like terms of both the expressions are grouped together and unlike terms are retained as it is.
- Sum of two or more like terms is a like term with a numerical coefficient equal to the sum of the numerical coefficients of all like terms.
- Difference between two like terms is a like term with a numerical coefficient equal to the difference between the numerical coefficients of the two like terms.
- For example, 2y + 3x − 2x + 4y
⇒ x(3−2) + y(2+4)
⇒ x+6y - Summation of algebraic expressions can be done in two ways:
Consider the summation of the algebraic expressions 5a2+7a+2ab and 7a2+9a+11b - Horizontal method
5a2+7a+2ab+7a2+9a+11b
= (5+7)a2+(7+9)a+2ab+11b
= 12a2+16a+2ab+11b - Vertical method
5a2+7a+2ab
7a2+9a+11b
¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯
12a2+16a+2ab+11b
Use of algebraic expressions in the formula of the perimeter of figures
Algebraic expressions can be used in formulating perimeter of figures.
Example: Let l be the length of one side then the perimeter of:
Equilateral triangle = 3l
Square = 4l
Regular pentagon = 5l
Use of algebraic expressions in formula of area of figures
Algebraic expressions can be used in formulation area of figures.
Example: Let l be the length and b be the breadth then the area of:
Square = l2
Rectangle = l×b = lb
Triangle = b×h/2, where b and h are base and height, respectively.