Introduction to Congruent Triangles
Congruent Figures
- Congruent figures are exactly equal in size and shape
Congruent Triangles
- If all the sides and angles of a triangle are equal to the corresponding sides and angles of another triangle, then both the triangles are said to be congruent.

Here, △ABC≅△DEF
Criteria for Congruency
SSS Criteria for Congruency
- If under a given correspondence, the three sides of one triangle are equal to the three corresponding sides of another triangle, then the triangles are congruent.

SAS Criteria for Congruency
- If under a correspondence, two sides and the angle included between them of a triangle are equal to two corresponding sides and the angle included between them of another triangle, then the triangles are congruent.

ASA Criteria for Congruency
- If under a correspondence, two angles and the included side of a triangle are equal to two corresponding angles and the included side of another triangle, then the triangles are congruent.

AAS Criteria for Congruency
AAS Rule: Triangles are congruent if two pairs of corresponding angles and a pair of opposite sides are equal in both triangles.


RHS Criteria for Congruency
- If under a correspondence, the hypotenuse and one side of a right-angled triangle are respectively equal to the hypotenuse and one side of another right-angled triangle, then the triangles are congruent.
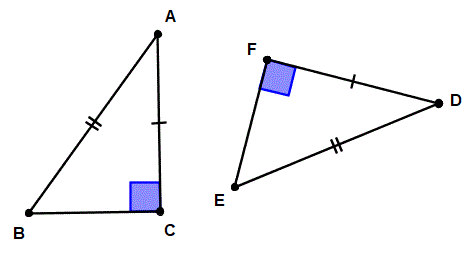
Criteria for Congruency
Criteria for Congruency of two triangles are:
(i) SSS Rule
(ii) SAS Rule
(iii) ASA Rule
(iv) RHS Rule