Average rate of reaction
The average rate of the reaction is the ratio of change in concentration of reactants to the change in time. It is determined by the change in concentration of reactants or products and the time taken for the change as well. As the reaction precedes forward the collisions between the molecules of the participating reactants reduces thereby decreasing the average rate of the reaction.
Mathematically, Average rate of reaction = Change in concentration / Time = (mol/litre)/time
PROBLEM. For the reaction R → P, the concentration of a reactant changes from 0.03 M to 0.02 M in 25 minutes. Calculate the average rate of reaction using units of time both in minutes and seconds.
SOLUTION. R2= 0.02 M
R1= 0.03M
t2 – t1= 25 minutes
∆[R]/∆t =∆[R2-R1]/ t2– t1=- (0.02-0.03)/25 = 6.67 X 10-6 Ms-1
=0.005ML-1 min-1
PROBLEM. In a reaction, 2A → Products, the concentration of A decreases from 0.5 mol L-1 to 0.4 mol L-1 in 10 minutes. Calculate the rate during this interval?
SOLUTION. -1/2 (∆[A]/∆t) =-1/2(∆[A2-A1]/∆t) =-1/2 (0.4-0.5/10)
=0.005ML-1 min-1
= 5 X 10-3M min-1
Instantaneous rate of reaction
The ratio of change in concentration in chemical reaction to the time period is termed as instantaneous rate of the reaction.
-d[R]/dt = change in chemical concentration over short period of time/ the short time elapsed = (mol/litre) / time
It can be calculated from the slope of the tangent on a concentration- time graph.
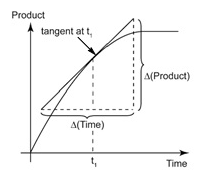
For example, consider the following graph.
The rate of reaction at t = 40s in the above graph can be calculated by following method:
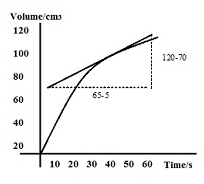
Rate of reaction = gradient of the tangent at 40s = (120-70)/(65-5) = 50/60= 0.83 cm3s-1
Rate expression
The representation of rate of reaction in terms of concentration of the reactants is called rate equation or rate expression.
For example, in the reaction
2NO(g) + O2(g) –> 2NO2
The rate expression is given as
Rate =k[NO]2[O2]
Let us consider another reaction
BrO3– + 5Br– + 6H+ –> 3Br + 3 H2O
Rate expression for this reaction is given as
k[BrO3–][Br–][H+]2
Order of a reaction
The addition of power of the concentration of reactant in a rate law expression gives the order of reaction.
Let A + 2B –> C + D be a chemical reaction.
From rate law R = k [A]x [B]y
Now Order of reaction is defined as addition of the order of all the reactants participating in a chemical reaction.
order w.r.t. A = x
Order w.r.t. B = y
Overall order of the given reaction = (x + y).
Units of order of reaction:
k = rate / [A]n = (mol.L-1s-1/molL-1) n
For 1st order reaction , n=1
k = rate / [A]1 = (mol.L-1s-1/molL-1) 1= s-1
For 2nd order reaction, n=2
k = rate / [A]2 = (mol.L-1s-1/molL-1) 2= L.mol-1s-1
PROBLEM. From the rate expression for the following reactions, determine their order of reaction and the dimensions of the rate constants.
(i) 3 NO(g) → N2O(g) Rate = k[NO]2
(ii) H2O2 (aq) + 3 I – (aq) + 2 H+→ 2 H2O (l) + I3– Rate = k[H2O2][I – ]
(iii) CH3CHO(g) → CH4(g) + CO(g) Rate = k [CH3CHO]3/2
(iv) C2H5Cl(g) → C2H4(g) + HCl(g) Rate = k [C2H5Cl]
SOLUTION. (i) Rate = k[NO]2
Order of the reaction = 2
Dimension of k = Rate / [NO]2
= mol L-1 s-1 / (mol L-1)2
= mol L-1 s-1 / mol2 L-2
= L mol-1 s-1
(ii) Rate = k[H2O2][I – ]
Order of the reaction = 2
Dimension of k = Rate / [H2O2][I – ]
= mol L-1 s-1 / (mol L-1) (mol L-1)
= L mol-1 s-1
(iii) Rate =k [CH3CHO]3/2
Order of reaction = 3/2
Dimension of k = Rate / [CH3CHO]3/2
= mol L-1 s-1 / (mol L-1)3/2
= mol L-1 s-1 / mol3/2 L-3/2
= L½ mol-½ s-1
(iv) Rate = k [C2H5Cl]
Order of the reaction = 1
Dimension of k = Rate / [C2H5Cl]
= mol L-1 s-1 / mol L-1
= s-1
PROBLEM. For a reaction, A + B → Product; the rate law is given by, r = k [A]½ [B]2. What is the order of the reaction?
SOLUTION. The order of the reaction = 1/2 + 2
= 2 1/2
= 2.5