Exercise 7.4 Page No: 171
1. Determine the ratio in which the line 2x + y – 4 = 0 divides the line segment joining the points A(2, –2) and B(3, 7).
Solution:
Consider line 2x + y – 4 = 0 divides line AB joined by the two points A(2, -2) and B(3, 7) in k : 1 ratio.
Coordinates of point of division can be given as follows:
x = and y =
Substituting the values of x and y given equation, i.e. 2x + y – 4 = 0, we have
2( + (– 4 = 0
+ ( = 4
4 + 6k – 2 + 7k = 4(k+1)
-2 + 9k = 0
Or k = 2/9
Hence, the ratio is 2:9.
2. Find the relation between x and y if the points (x, y), (1, 2) and (7, 0) are collinear.
Solution:
If given points are collinear then area of triangle formed by them must be zero.
Let (x, y), (1, 2) and (7, 0) are vertices of a triangle,
Area of a triangle = = 0
[x(2 – 0) + 1 (0 – y) + 7( y – 2)] = 0
- 2x – y + 7y – 14 = 0
- 2x + 6y – 14 = 0
- x + 3y – 7 = 0. Which is required result.
3. Find the centre of a circle passing through points (6, -6), (3, -7) and (3, 3).
Solution:
Let A = (6, -6), B = (3, -7), C = (3, 3) are the points on a circle.
If O is the centre, then OA = OB = OC (radii are equal)
If O = (x, y) then
OA =
OB =
OC =
Choose: OA = OB, we have
After simplifying above, we get -6x = 2y – 14 ….(1)
Similarly: OB = OC
(x – 3)2 + (y + 7)2 = (x – 3)2 + (y – 3)2
(y + 7)2 = (y – 3)2
y2 + 14y + 49 = y2 – 6y + 9
20y =-40
or y = -2
Substituting the value of y in equation (1), we get;
-6x = 2y – 14
-6x = -4 – 14 = -18
x = 3
Hence, centre of the circle located at point (3,-2).
4. The two opposite vertices of a square are (-1, 2) and (3, 2). Find the coordinates of the other two vertices.
Solution:
Let ABCD is a square, where A(-1,2) and B(3,2). And Point O is the point of intersection of AC and BD
To Find: Coordinate of points B and D.
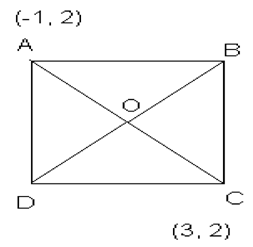
Step 1: Find distance between A and C and coordinates of point O.
We know that, diagonals of a square are equal and bisect each other.
AC = = 4
Coordinates of O can be calculated as follows:
x = (3-1)/2 = 1 and y = (2+2)/2 = 2
So O(1,2)
Step 2: Find the side of the square using Pythagoras theorem
Let a be the side of square and AC = 4
From right triangle, ACD,
a = 2√2
Hence, each side of square = 2√2
Step 3: Find coordinates of point D
Equate length measure of AD and CD
Say, if coordinate of D are
AD =
Squaring both sides,
AD2 =
Similarly, CD2 =
Since all sides of a square are equal, which means AD = CD
Value of y1 can be calculated as follows by using the value of x.
From step 2: each side of square = 2√2
CD2 =
8 =
Hence, D = (1, 4)
Step 4: Find coordinates of point B
From line segment, BOD
Coordinates of B can be calculated using coordinates of O; as follows:
Earlier, we had calculated O = (1, 2)
Say B =
For BD;
1 =
x2 = 1
And 2 =
=> y2 = 0
Therefore, the coordinates of required points are B = (1,0) and D = (1,4)
5. The class X students of a secondary school in Krishinagar have been allotted a rectangular plot of land for their gardening activity. Saplings of Gulmohar are planted on the boundary at a distance of 1 m from each other. There is a triangular lawn in the plot as shown in the fig. 7.14. The students are to sow the seeds of flowering plants on the remaining area of the plot.
(i) Taking A as origin, find the coordinates of the vertices of the triangle.
(ii) What will be the coordinates of the vertices of triangle PQR if C is the origin?
Also calculate the areas of the triangles in these cases. What do you observe?

Solution:
(i) Taking A as origin, coordinates of the vertices P, Q and R are,
From figure: P = (4, 6), Q = (3, 2), R (6, 5)
Here AD is the x-axis and AB is the y-axis.
(ii) Taking C as origin,
Coordinates of vertices P, Q and R are ( 12, 2) , (13, 6) and (10, 3) respectively.
Here CB is the x-axis and CD is the y-axis.
Find the area of triangles:
Area of triangle PQR in case of origin A:
Using formula: Area of a triangle =
= ½ [4(2 – 5) + 3 (5 – 6) + 6 (6 – 2)]
= ½ ( – 12 – 3 + 24 )
= 9/2 sq unit
(ii) Area of triangle PQR in case of origin C:
Area of a triangle =
= ½ [ 12(6 – 3) + 13 ( 3 – 2) + 10( 2 – 6)]
= ½ ( 36 + 13 – 40)
= 9/2 sq unit
This implies, Area of triangle PQR at origin A = Area of triangle PQR at origin C
Area is same in both case because triangle remains the same no matter which point is considered as origin.
6. The vertices of a ∆ ABC are A (4, 6), B (1, 5) and C (7, 2). A line is drawn to intersect sides AB and AC at D and E respectively, such that . Calculate the area of the ∆ ADE and compare it with area of ∆ ABC. (Recall Theorem 6.2 and Theorem 6.6)
Solution:
Given: The vertices of a ∆ ABC are A (4, 6), B (1, 5) and C (7, 2)

Point D and Point E divide AB and AC respectively in ratio 1 : 3.
Coordinates of D can be calculated as follows:
x = and y =
Here
Consider line segment AB which is divided by the point D at the ration 1:3.
x =
y =
Similarly, Coordinates of E can be calculated as follows:
x =
y = = 5
Find Area of triangle:
Using formula: Area of a triangle =
Area of triangle ∆ ABC can be calculated as follows:
= ½ [4(5 – 2) + 1( 2 – 6) + 7( 6 – 5)]
= ½ (12 – 4 + 7) = 15/2 sq unit
Area of ∆ ADE can be calculated as follows:
= ½ [4(23/4 – 5) + 13/4 (5 – 6) + 19/4 (6 – 23/4)]
= ½ (3 – 13/4 + 19/16)
= ½ ( 15/16 ) = 15/32 sq unit
Hence, ratio of area of triangle ADE to area of triangle ABC = 1 : 16.
7. Let A (4, 2), B (6, 5) and C (1, 4) be the vertices of ∆ ABC.
(i) The median from A meets BC at D. Find the coordinates of point D.
(ii) Find the coordinates of the point P on AD such that AP : PD = 2 : 1.
(iii) Find the coordinates of point Q and R on medians BE and CF respectively such that BQ : QE = 2:1 and CR : RF = 2 : 1.
(iv) What do you observe?
[Note : The point which is common to all the three medians is called the centroid
and this point divides each median in the ratio 2 : 1.](v) If A (x1, y1), B (x2, y2) and C (x3, y3) are the vertices of triangle ABC, find the coordinates of the centroid of the triangle.
Solution:
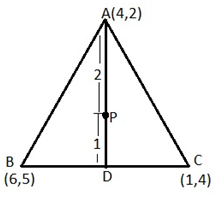
(i) Coordinates of D can be calculated as follows:
Coordinates of D =
D
(ii) Coordinates of P can be calculated as follows:
Coordinates of P =
P
(iii) Coordinates of E can be calculated as follows:
Coordinates of E = = (5/2 , 3)
E(5/2 , 3)
Point Q and P would be coincident because medians of a triangle intersect each other at a common point called centroid. Coordinate of Q can be given as follows:
Coordinates of Q =
F is the mid- point of the side AB
Coordinates of F =
Point R divides the side CF in ratio 2:1
Coordinates of R =
(iv) Coordinates of P, Q and R are same which shows that medians intersect each other at a common point, i.e. centroid of the triangle.
(v) If A (x1, y1), B (x2, y2) and C (x3, y3) are the vertices of triangle ABC, the coordinates of centroid can be given as follows:
8. ABCD is a rectangle formed by the points A (-1, – 1), B (-1, 4), C (5, 4) and D (5, -1). P, Q, R and S are the midpoints of AB, BC, CD and DA respectively. Is the quadrilateral PQRS a square? a rectangle? or a rhombus? Justify your answer.
Solution:
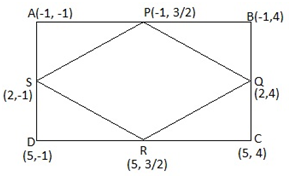
P id the mid-point of side AB,
Coordinate of P =
Similarly, Q, R and S are (As Q is mid-point of BC, R is midpoint of CD and S is midpoint of AD)
Coordinate of Q = (2, 4)
Coordinate of R = (5,
Coordinate of S = (2, -1)
Now,
Length of PQ = = =
Length of SP = = =
Length of QR = = =
Length of RS = = =
Length of PR (diagonal) = = 6
Length of QS (diagonal) = = 5
The above values show that, PQ = SP = QR = RS = , i.e. all sides are equal.
But PR ≠ QS i.e. diagonals are not of equal measure.
Hence, the given figure is a rhombus.