Exercise 13.6 Page No: 230

1. The circumference of the base of cylindrical vessel is 132cm and its height is 25cm.

How many litres of water can it hold? (1000 cm³= 1L) (Assume π = 22/7)

Solution:

Circumference of the base of cylindrical vessel = 132 cm

Height of vessel, h = 25 cm

Let r be the radius of the cylindrical vessel.

Step 1: Find the radius of vessel

We know that, circumference of base = 2πr, so

2πr = 132 (given)

r = (132/(2 π))

r = 66×7/22 = 21

Radius is 21 cm

Step 2: Find the volume of vessel

Formula: Volume of cylindrical vessel = πr²h

= (22/7)×21²×25

= 34650

Therefore, volume is 34650 cm³

Since, 1000 cm³ = 1L

So, Volume = 34650/1000 L= 34.65L

Therefore, vessel can hold 34.65 litres of water.

2. The inner diameter of a cylindrical wooden pipe is 24cm and its outer diameter is 28 cm. The length of the pipe is 35cm.Find the mass of the pipe, if 1cm³ of wood has a mass of 0.6g. (Assume π = 22/7)

Solution:

Inner radius of cylindrical pipe, say r₁ = diameter₁/ 2 = 24/2 cm = 12cm

Outer radius of cylindrical pipe, say r₂ = diameter₂/ 2 = 28/2 cm = 14 cm

Height of pipe, h = Length of pipe = 35cm

Now, the Volume of pipe = π(r₂²-r₁²)h cm³

Substitute the values.

Volume of pipe = 110×52 cm³ = 5720 cm³

Since, Mass of 1 cm³ wood = 0.6 g

Mass of 5720 cm³ wood = (5720×0.6) g = 3432 g or 3.432 kg. Answer!

3. A soft drink is available in two packs – (i) a tin can with a rectangular base of length 5cm and width 4cm, having a height of 15 cm and (ii) a plastic cylinder with circular base of diameter 7cm and height 10cm. Which container has greater capacity and by how much? (Assume π=22/7)

Solution:

1. tin can will be cuboidal in shape

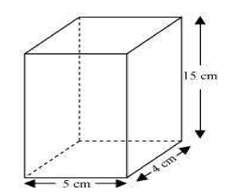
Dimensions of tin can are

Length, l = 5 cm

Breadth, b = 4 cm

Height, h = 15 cm

Capacity of tin can = l×b×h= (5×4×15) cm³ = 300 cm³

1. Plastic cylinder will be cylindrical in shape.

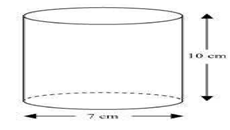
Dimensions of plastic can are:

Radius of circular end of plastic cylinder, r = 3.5cm

Height , H = 10 cm

Capacity of plastic cylinder = πr²H

Capacity of plastic cylinder = (22/7)×(3.5)²×10 = 385

Capacity of plastic cylinder is 385 cm³

From results of (i) and (ii), plastic cylinder has more capacity.

Difference in capacity = (385-300) cm³ = 85cm³

4. If the lateral surface of a cylinder is 94.2cm² and its height is 5cm, then find

(i) radius of its base (ii) its volume.[Use π= 3.14]

Solution:

CSA of cylinder = 94.2 cm²

Height of cylinder, h = 5cm

(i) Let radius of cylinder be r.

Using CSA of cylinder, we get

2πrh = 94.2

2×3.14×r×5 = 94.2

r = 3

radius is 3 cm

(ii) Volume of cylinder

Formula for volume of cylinder = πr²h

Now, πr²h = (3.14×(3)²×5) (using value of r from (i))

= 141.3

Volume is 141.3 cm³

5. It costs Rs 2200 to paint the inner curved surface of a cylindrical vessel 10m deep. If the cost of painting is at the rate of Rs 20 per m², find

(i) inner curved surface area of the vessel

(ii) radius of the base

(iii) capacity of the vessel

(Assume π = 22/7)

Solution:

(i) Rs 20 is the cost of painting 1 m² area.

Rs 1 is the cost to paint 1/20 m² area

So, Rs 2200 is the cost of painting = (1/20×2200) m²

= 110 m² area

The inner surface area of the vessel is 110m².

(ii) Radius of the base of the vessel, let us say r.

Height (h) = 10 m and

Surface area formula = 2πrh

Using result of (i)

2πrh = 110 m²

2×22/7×r×10 = 110

r = 1.75

Radius is 1.75 m.

(iii) Volume of vessel formula = πr²h

Here r = 1.75 and h = 10

Volume = (22/7)×(1.75)²×10 ) = 96.25

Volume of vessel is 96.25 m³

Therefore, the capacity of the vessel is 96.25 m³ or 96250 litres.

6. The capacity of a closed cylindrical vessel of height 1m is15.4 liters. How many square meters of metal sheet would be needed to make it? (Assume π = 22/7)

Solution:

Height of cylindrical vessel, h = 1 m

Capacity of cylindrical vessel = 15.4 litres = 0.0154 m³

Let r be the radius of the circular end.

Now,

Capacity of cylindrical vessel = (22/7)×r²×1) = 0.0154

After simplifying, we get, r = 0.07 m

Again, total surface area of vessel = 2πr(r+h)

= (2×22/7×0.07(0.07+1))

= 0.44×1.07

= 0.4708

Total surface area of vessel is 0.4708 m²

Therefore, 0.4708 m² of the metal sheet would be required to make the cylindrical vessel.

7. A lead pencil consists of a cylinder of wood with solid cylinder of graphite filled in the interior. The diameter of the pencil is 7 mm and the diameter of the graphite is 1 mm. If the length of the pencil is 14 cm, find the volume of the wood and that of the graphite. (Assume π = 22/7)

Solution:

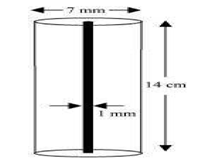
Radius of pencil, r₁ = 7/2 mm = 0.7/2 cm = 0.35 cm

Radius of graphite, r₂ = 1/2 mm = 0.1/2 cm = 0.05 cm

Height of pencil, h = 14 cm

Formula to find, volume of wood in pencil = (r₁²-r₂²)h cubic units

Substitute the values, we have

= [(22/7)×(0.35²-0.05²)×14]

= 44×0.12

= 5.28

This implies, volume of wood in pencil = 5.28 cm³

Again,

Volume of graphite = r₂²h cubic units

Substitute the values, we have

= (22/7)×0.05²×14

= 44×0.0025

= 0.11

So, the volume of graphite is 0.11 cm³.

8. A patient in a hospital is given soup daily in a cylindrical bowl of diameter 7cm. If the bowl is filled with soup to a height of 4cm, how much soup the hospital has to prepare daily to serve 250 patients? (Assume π = 22/7)

Solution:

Diameter of cylindrical bowl = 7 cm

Radius of cylindrical bowl, r = 7/2 cm = 3.5 cm

Bowl is filled with soup to a height of4cm, so h = 4 cm

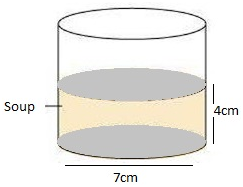
Volume of soup in one bowl= πr²h

(22/7)×3.5²×4 = 154

Volume of soup in one bowl is 154 cm³

Therefore,

Volume of soup given to 250 patients = (250×154) cm³= 38500 cm³

= 38.5litres. Answer!