Important Questions & Solutions for Class 9 Chapter 3 (Coordinate Geometry)
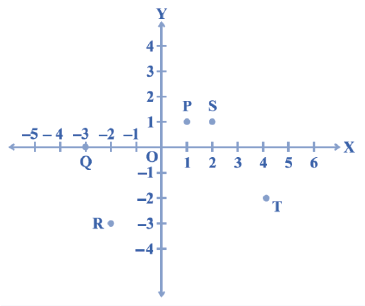
1. Write the coordinates of each of the points P, Q, R, S, T and O from the figure given.
Solution:
The coordinates of the points P, Q, R, S, T and O are as follows:
P = (1, 1)
Q = (-3, 0)
R = (-2, -3)
S = (2, 1)
T = (4, -2)
O = (0, 0)
Q.2: Plot the following points and check whether they are collinear or not:
(i) (1, 3), (– 1, – 1), (– 2, – 3)
(ii) (1, 1), (2, – 3), (– 1, – 2)
(iii) (0, 0), (2, 2), (5, 5)
Solution:
(i)
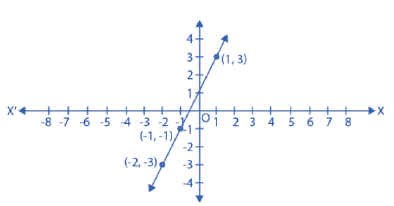
The points (1, 3), (– 1, – 1), (– 2, – 3) lie in a straight line,
Hence, the points are collinear.
(ii)
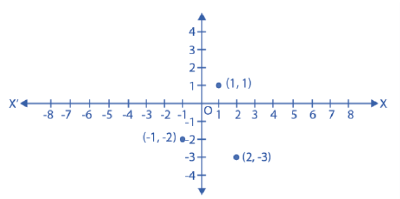
The points (1, 1), (2, – 3), (– 1, – 2) do not lie in a straight line,
Hence, the points are not collinear.
(iii)
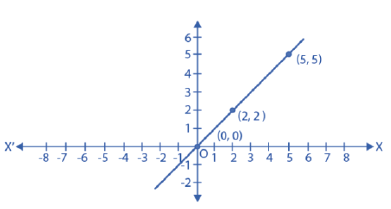
The points (0, 0), (2, 2), (5, 5) lie in a straight line,
Hence, the points are collinear.
Q.3: Without plotting the points indicate the quadrant in which they will lie, if
(i) ordinate is 5 and abscissa is – 3
(ii) abscissa is – 5 and ordinate is – 3
(iii) abscissa is – 5 and ordinate is 3
(iv) ordinate is 5 and abscissa is 3
Solution:
(i) The point is (-3,5).
Hence, the point lies in the II quadrant.
(ii) The point is (-5,-3).
Hence, the point lies in the III quadrant.
(iii) The point is (-5,3).
Hence, the point lies in the II quadrant.
(iv) The point is (3,5).
Hence, the point lies in the I quadrant.
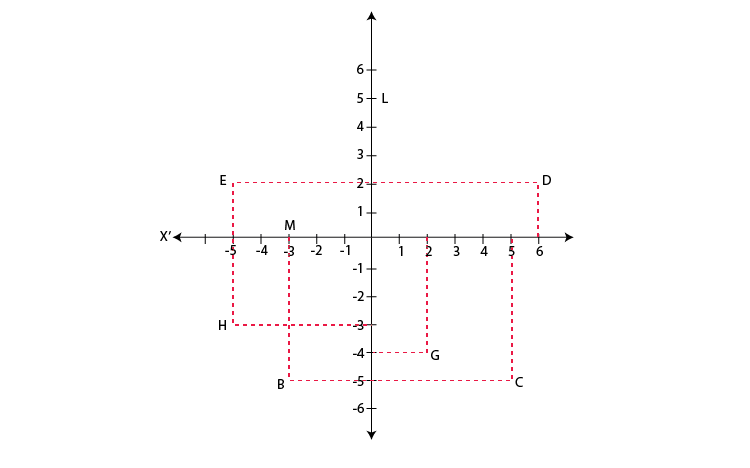
Q.4: See figure and write the following:
- The coordinates of B.
- The coordinates of C.
- The point identified by the coordinates (–3, –5).
- The point identified by the coordinates (2, – 4).
- The abscissa of the point D.
- The ordinate of the point H.
- The coordinates of the point L.
- The coordinates of the point M.
Solution:
- The co-ordinates of B is (−5, 2).
- The co-ordinates of C is (5, −5).
- The point identified by the coordinates (−3, −5) is E.
- The point identified by the coordinates (2, −4) is G.
- Abscissa means x co-ordinate of point D. So, abscissa of the point D is 6.
- Ordinate means y coordinate of point H. So, the ordinate of point H is -3.
- The coordinates of the point L is (0, 5).
- The coordinates of the point M is (−3, 0).
Q.5: Plot the points (x, y) given in the following table on the plane, choosing suitable units of distance on the axes.
| x | -2 | -1 | 0 | 1 | 3 |
| y | 8 | 7 | -1.25 | 3 | -1 |
Solution:
The points to plotted on the(x,y) are:
- (-2,8)
- (-1,7)
- (0,-1.25)
- (1,3)
- (3,-1)
On the graph mark X-axis and Y-axis. Mark the meeting point as O.
Now, Let 1 unit = 1 cm
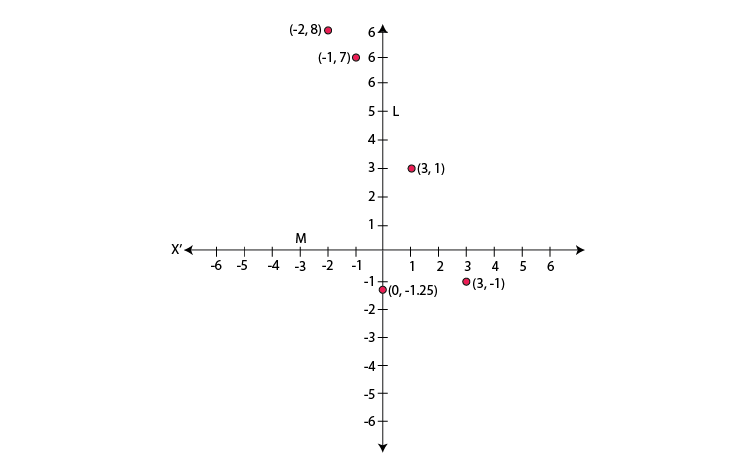
- (-2,8): II- Quadrant, Meeting point of the imaginary lines that starts from 2 units to the left of origin O and from 8 units above the origin O
- (-1,7): II- Quadrant, Meeting point of the imaginary lines that starts from 1 units to the left of origin O and from 7 units above the origin O
- (0,-1.25): On the y-axis, 1.25 units to the bottom of origin O
- (1,3): I- Quadrant, Meeting point of the imaginary lines that starts from 1 units to the right of origin O and from 3 units above the origin O
- (3,-1): IV- Quadrant, Meeting point of the imaginary lines that starts from 3 units to the right of origin O and from 1 unit below the origin O
Q.6: Write the answer to each of the following questions:
(i) What is the name of the horizontal and the vertical lines drawn to determine the position of any point in the Cartesian plane?
(ii) What is the name of each part of the plane formed by these two lines?
(iii) Write the name of the point where these two lines intersect
Solution:
(i) The name of horizontal and vertical lines drawn to determine the position of any point in the Cartesian plane is x-axis and y-axis respectively.
(ii) The name of each part of the plane formed by these two lines x-axis and the y-axis is quadrants.
(iii) The point where these two lines intersect is called the origin
Extra Questions For Class 9th Maths Chapter 3
- Points A (5, 3), B (–2, 3) and D (5, –4) are three vertices of a square ABCD. Plot these points on a graph paper and hence find the coordinates of the vertex C.
- Write the coordinates of the vertices of a rectangle whose length and breadth are 5 and 3 units respectively, one vertex at the origin, the longer side lies on the x-axis and one of the vertices lies in the third quadrant.
- Plot the points (x, y) given by the following table:
| x | 2 | 4 | -3 | -2 |
| y | 4 | 2 | 0 | 5 |
4. Plot the following points and write the name of the figure obtained by joining them in order:
P(– 3, 2), Q (– 7, – 3), R (6, – 3), S (2, 2)
5. Locate the points (5, 0), (0, 5), (2, 5), (5, 2), (–3, 5), (–3, –5), (5, –3) and (6, 1) in the Cartesian plane.