What is Euclid’s Geometry?
The word “geometry” comes from the Greek words “geo”, which means the “earth”, and “metron”, which means “to measure”. Euclidean geometry is a mathematical system attributed to Euclid a teacher of mathematics in Alexandria in Egypt. Euclid gave us an exceptional idea regarding the basic concepts of geometry, in his book called “Elements”.
Euclid listed 23 definitions in his book “Elements”. Some important points are mentioned below:
- A line is an endless length.
- A point has no dimension (length, breadth and width).
- A line which lies evenly with the points on itself is a straight line.
- Points are the ends of a line.
- A surface is that which has breadth and length only.
- A plane surface is a surface which lies evenly with the straight lines on itself.
- Lines are the edges of a surface.
Euclid realized that a precise development of geometry must start with the foundations. Euclid’s axioms and postulates are still studied for a better understanding of geometry.
Important Questions
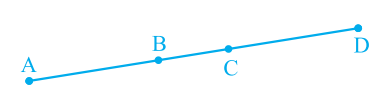
Q.1) If A, B and C are 3 points on a line, and B lies between A and C, prove that AB + BC = AC.
Q.2) Prove that an equilateral triangle can be formed on any given line segment.
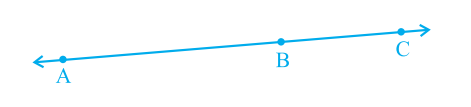
Q.3) If AC = BD, then prove that AB = CD.