Linear Momentum of System of Particles

• The total momentum of a system of particles is equal to the product of the total mass of the system and the velocity of its COM.
• P = p₁ + p₂ + …. + p_n

          = m₁v₁ + m₂v₂ + …. + m_nv_n

• P = MV

      Where

• pi = momentum of i^th particle ,
• P = momentum of system of particles ,
• V = velocity of COM

• Newton’s Second Law extended to system of particles: dP/dt = F_ext .
• When the total external force acting on a system of particles is zero (F_ext = 0), the total linear momentum of the system is constant (dP/dt = 0 => P = constant). Also the velocity of the centre of mass remains constant (Since P = mv = Constant ).
• If the total external force on a body is zero, then internal forces can cause complex trajectories of individual particles but the COM moves with a constant velocity.
• Example: Decay of Ra atom into He atom & Rn Atom
• Case I – If Ra atom was initially at rest, He atom and Rn atom will have opposite direction of velocity, but the COM will remain at rest.

Example – A bullet of mass m is fired at a velocity of v1, and embeds itself in a block of mass M, initially at rest and on a frictionless surface. What is the final velocity of the block?

Solution: Now if we take bullet and block as a system, then no external force is acting on it. So we can conserve momentum. 
(m₁v₁ + Mv₂)_before = (m₁+ M)v_after
v_after =  m₁v₁/( m₁+ M) , as the initial velocity of block M is zero.

Vector Product

• Vector product (cross product) of two vectors a and b is a × b = ab sinΘ  = c ,     where Θ is angle between a & b
• Vector product c is perpendicular to the plane containing a and b.
• If you keep your palm in direction of vector a and curl your fingers to the direction a to b, your thumb will give you the direction of vector product c

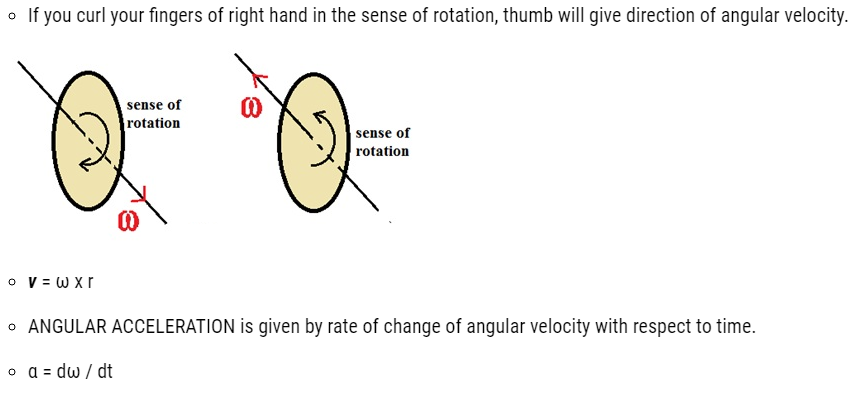
Angular velocity & its relation with linear velocity

Every particle of a rotating body moves in a circle. Angular displacement of a given particle about its centre in unit time is defined as angular velocity.