Stress:-

• Stress is the restoring force per unit area.
• Whenever we apply an external force on the body to change its shape there is a restoring force that develops in the body in the opposite direction.
• For example:-
  • When we apply an external force to a rubber ball at the same instant of time some force develops in the ball which acts in the opposite direction.
  • This opposite force which develops in the ball when an external force is applied is known as restoring force.
  • Both the forces are equal in magnitude.

• Mathematically:-
• Stress = F/A  
• Where F= restoring force develops in the body because of force we apply.
• A=area 
• S.I. Unit :- N/m² or Pascal(Pa)  
• Dimensional formula is [ML^–1T^–2].

Types of Stress: Longitudinal stress

• Longitudinal stress is defined as restoring force per unit area when the force is applied to the cross-sectional area of the cylindrical body. 
• Consider a cylinder which we have to deform. If we apply the force perpendicular to the cross-sectional area, there will be a restoring force that develops in the cylinder in the opposite direction. 
• This restoring force per unit area is known as longitudinal stress. 
• Experimentally we can observe the increase in length. 
• If we tie a heavy object to the cylinder with the help of threads. 
• Let Initial length of the cylinder is L. 
• After it gets stretched its length increases by ΔL due to the stress. 
• As there is change in the length therefore this type of stress is known as longitudinal stress. 
• In the below figure if we attach a box to the cylinder, a force is applied on the cross-sectional area of cylinder due to which it gets stretched and as a result there is change in the length of the cylinder.

Problem:- A 14.5 kg mass, fastened to the end of a steel wire of unstretched length 1.0 m, is whirled in a vertical circle with an angular velocity of 2 rev/s at the bottom of the circle. The cross-sectional area of the wire is 0.065 cm². Calculate the elongation of the wire when the mass is at the lowest point of its path.

Answer:- Mass, m = 14.5 kg

Length of the steel wire, l = 1.0 m

Angular velocity, ω = 2 rev/s

Cross-sectional area of the wire, a = 0.065 cm²

Let Δl be the elongation of the wire when the mass is at the lowest point of its path.

When the mass is placed at the position of the vertical circle, the total force on the mass is:

F = mg + mlω²

= 14.5 × 9.8 + 14.5 × 1 × (2)² = 200.1 N

Young’s modulus =Stress/Strain

Y = (F/A)/ Δl/l

= (F l)/A Δl

Therefore Δl = F l/A Y

Young’s modulus for steel = 2 × 10¹¹ Pa

Therefore Δl = 200.1/0.065×10^-4x2x10¹¹ = 1539.23×10⁷

=1.539×10^-4m

Hence, the elongation of the wire is 1.539 × 10^–4 m.

Types of Longitudinal Stress:-

1. Tensile Stress
2. Compressive Stress

Tensile Stress

• Tensile stress is a longitudinal stress when the length of the cylinder increases.

For example:-

• When the force is applied to both sides of the cylinder, the cylinder gets stretched. As a result there will be increase in its length.

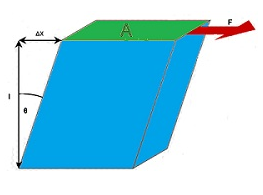
Tangential or Shearing Stress

• Restoring force per unit area when the force applied is parallel to the cross sectional area of the body.
• Relative displacement occurs between the opposite faces of the body.
• For example:-

• Consider a cube. If we apply force parallel to the cross sectional area there will be movement which takes place between the opposite faces of the cube as they have relative motion with each other.
• This type of stress is known as tangential or shearing stress.

Hydraulic Stress

• Hydraulic stress is the restoring force per unit area when force is applied by a fluid on the body.
• For example:-

1. Consider a rubber ball and if it is dipped in the pond .Due to the pressure of water from all directions force acts on the ball as a result, the ball seems to be slightly contracted.
2. Because of the force exerted by the water there is restoring force which develops in the ball which is equal in magnitude to the force applied by the water but in opposite direction.
3. This type of stress is known as hydraulic stress.