Viscosity

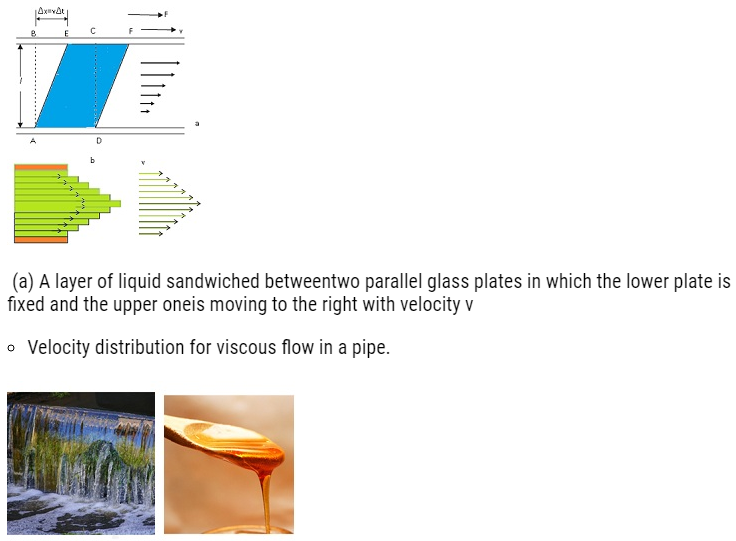
• Viscosity is the property of a fluid that resists the force tending to cause the fluid to flow.
• It is analogous to friction in solids.
• Example:-
  • Consider 2 glasses one filled with water and the other filled with honey.
  • Water will flow down the glass very rapidly whereas honey won’t.This is because honey is more viscous than water.
  • Therefore in order to make honey flow we need to apply greater amount of force. Because honey has the property to resist the motion.
• Viscosity comes into play when there is relative motion between the layers of the fluid.The different layers are not moving at the same pace.

Coefficient of Viscosity

• Coefficient of viscosity is the measure of degree to which a fluid resists flow under an applied force.
• This means how much resistance does a fluid have to its motion.
• Ratio of shearing stress to the strain rate.
• It is denoted by ‘η’.
• Mathematically
  • Δt=time , displacement =Δx
  • Therefore,
    • shearing stress =Δx/l where l= length
    • Strain rate=Δx/lΔt
  • η=shearing stress/strain rate
    • (F/A)/(Δx/lΔt) = (Fl)/vA where Δx/t=v
  • Therefore η=(Fl)/ vA
  • I. Unit:- Poiseiulle (PI)/Pa/Nsm^-2
  • Dimensional Formula: [ML^-1T^-1]

Answer:-

The metal block moves to the rightbecause of the tension in the string. The tension

T is equal in magnitude to the weight of thesuspended mass m. Thus the shear force F is

F = T = mg = 0.010 kg × 9.8 ms^–2 = 9.8 × 10^-2 N

Shear stress on the fluid = F/A =9.8 x10^–2/0.10

Strain rate =v/l =0.085/0.030

η= stress/strain rate

= (9.8×10^-2x 0.30 x10^-3m)/ (0.085ms^-1x0.10m²)

= 3.45 × 10^-3 Pa s

Stokes Law

• The force that retards a sphere moving through a viscous fluid is directly ∝to the velocity and the radius of the sphere, and the viscosity of the fluid.
• Mathematically:-F =6πηrv where
  • Let retarding force F∝v where v =velocity of the sphere
    • F ∝ r where r=radius of the sphere
    • F∝η where η=coefficient of viscosity
    • 6π=constant
  • Stokes law is applicable only to laminar flow of liquids.It is not applicable to turbulent law.
  • Example:-Falling raindrops
    • Consider a single rain drop, when rain drop is falling it is passing through air.
    • The air has some viscosity; there will be some force which will try to stop the motion of the rain drop.
    • Initially the rain drop accelerates but after some time it falls with constant velocity.
    • As the velocity increases the retarding force also increases.
    • There will be viscous force F_v and bind force F_bacting in the upward direction.There will also be F_ggravitational force acting downwards.
    • After some time F_g = F_r (F_v+F_b)
    • Net Force is 0. If force is 0 as a result acceleration also becomes 0.

Terminal Velocity

• Terminal velocity is the maximum velocity of a body moving through a viscous fluid.
• It is attained when force of resistance of the medium is equal and opposite to the force of gravity.
• As the velocity is increasing the retarding force will also increase and a stage will come when the force of gravity becomes equal to resistance force.
• After that point velocity won’t increase and this velocity is known as terminal velocity.
• It is denoted by ‘v_t’.Where_t=terminal.
• Mathematically:-
  • Terminal velocity is attained when Force of resistance = force due to gravitational attraction.
  • 6πηrv =mg
  • 6πηrv = densityxVg (Because density=m/V), density=ρ – σ where ρ and σ are the densities of the sphere and the viscous medium resp.
  • 6πηrv = (ρ – σ)x4/3πr³g where Volume of the sphere(V) =4/3πr³
  • By simplifying
    • =(ρ – σ)gx4/3r²x1/(6η)
  • v_t =2r²(ρ – σ)g/9 η .This is the terminal velocity. Where(v=v_t)

Problem: The terminal velocity of acopper ball of radius 2.0 mm fallingthrough a tank of oil at 20^oC is 6.5 cm s^-1.Compute the viscosity of the oil at 20^oC.Density of oil is 1.5 × 10³ kg m^-3, density of

copper is 8.9 × 10³ kg m^-3.

Answer:-

Given: – v_t = 6.5 × 10^-2 ms^-1, a = 2 × 10^-3 m,g = 9.8 ms^-2, ρ = 8.9 × 10³ kg m^-3,

σ =1.5 × 10³ kg m^-3. From Equation: -v_t =2r² (ρ – σ) g/9 η

=2/9(2 x10^-3 m² x 9.8ms^-2/ 6.5×10^-2ms^-1) x 7.4 10³ kgm^-3

=9.9 x 10^-1 kgm^-1s^-1

Problem: Calculate the terminal velocity in air of an oil drop of radius 2×10⁵ m from the following data g=9.8m/s²; coefficient of viscosity of air =1.8×10^-5Pas; density of oil=900kg/m³.The up thrust of air may be neglected?

Answer:

Radius r =2×10^-5 m

g=9.8m/s²

η=1.8×10^-5Pas

ρ =900kg/m³

v=v_twhen 6πηrv = mg

6πηrv =ρx4/3r³g

Simplifying: – v_t =2r²gρ/9π = (2 x (2×10^-5)²x9.8×900)/9×1.8×10^-5

=4.36cm/s