Surface Tension
- Surface tension is the property of the liquid surface which arises due to the fact that surface molecules have extra energy.
- Surface energy is the extra energy which the molecules at the surface have.
- Surface tension is the property of the liquid surface because the molecules have extra energy.
- Surface energy is defined as surface energy per unit area of the liquid surface.
- Denoted by ’S’.
- Mathematically :-
- Consider a case in which liquid is enclosed in a movable bar.
- Slide the bar slightly and it moves some distance (‘d’).
- There will be increase in the area, (dl) where l=length of the bar.
- Liquids have two surfaces one on the bar and other above the bar. Therefore area=2(dl)
- Work done for this change =Fxdisplacement.
- Surface tension(S)=Surface Energy/area
- Or Surface Energy=S x area
- =Sx2dl
- Therefore S x 2dl =F x d
- S = F/2d
- Surface tension is the surface energy per unit area of the liquid surface.
- It can be also defined as Force per unit length on the liquid surface.
- Important: -At any interface (it is a line which separates two different medium) the surface tension always acts in equal and opposite direction and it is always perpendicular to the line at the interface.

Surface tension and Surface energy: practical applications
- Consider a molecule which is present completely inside the liquid and if it is strongly attracted by the neighbouring molecules then the surface energy is less.
- Consider a molecule which is present partially inside the liquid the force of attraction by the neighbouring molecules is lesser as a result surface energy is more.
- Consider a molecule whose very little part is inside the water so very small force of attraction by the neighbouring molecules as a result more surface energy.
- Conclusion: – A fluid will stick to a solid surface if the surface energy between fluid and solid is smaller than the sum of energies between solid-air and fluid-air.
- This means Ssf( solid fluid) < Sfa(fluid air) + Ssa(Solid air)


How detergents work?
- Washing alone with the water can remove some of the dirt but it does not remove the grease stains. This is because water does not wet greasy dirt.
- We need detergent which mixes water with dirt to remove it from the clothes.
- Detergent molecules look like hairpin shape. When we add detergents to the water one end stick to water and the other end sticks to the dirt.
- As a result dirt is getting attracted to the detergent molecules and they get detached from the clothes and they are suspended in the water.
- Detergent molecules get attracted to water and when water is removed the dirt also gets removed from the clothes.

Detergent action in terms of whatdetergent molecules do.
In image (1) Soap molecules with head attracted to water
In image (2) greasy dirt
In image (3) water is added but dirt does not get removed
In image (4) when detergent is added, other end of the molecules get attracted to the boundary where water meets dirt.
In image (5) Dirt gets surrounded by inert end and dirt from the clothes can be removed by moving water.
In image (6) dirt is held suspended, surrounded by soap molecule,
Angle of Contact
- Angle of contact is the angle at which a liquid interface meets a solid surface.
- It is denoted by θ.
- It is different at interfaces of different pairs of liquids and solids.
- For example: – Droplet of water on louts leaf. The droplet of water(Liquid) is in contact with the solid surface which is leaf.
- This liquid surface makes some angle with the solid surface.This angle is known as angle of contact.

Significance of Angle of Contact
- Angle of contact determines whether a liquid will spread on the surface of a solid or it will form droplets on it.
- If the Angle of contact is obtuse:then droplet will be formed.
- If the Angle of contact is acute: then the water will spread.
- Case1: When droplet is formed
- Consider we have a solid surface, droplet of water which is liquid and air.
- The solid liquid interface denoted by Ssl, solid air interface denoted by Ssaand liquid air interface denoted by Sla.
- The angle which Ssl makes with Sla. It is greater than the 900.
- Therefore droplet is formed.

Drops and Bubbles
Why water and bubbles are drops?
- Whenever liquid is left to itself it tends to acquire the least possible surface area so that it has least surface energy so it has most stability.
- Therefore for more stability they acquire the shape of sphere, as sphere has least possible area.



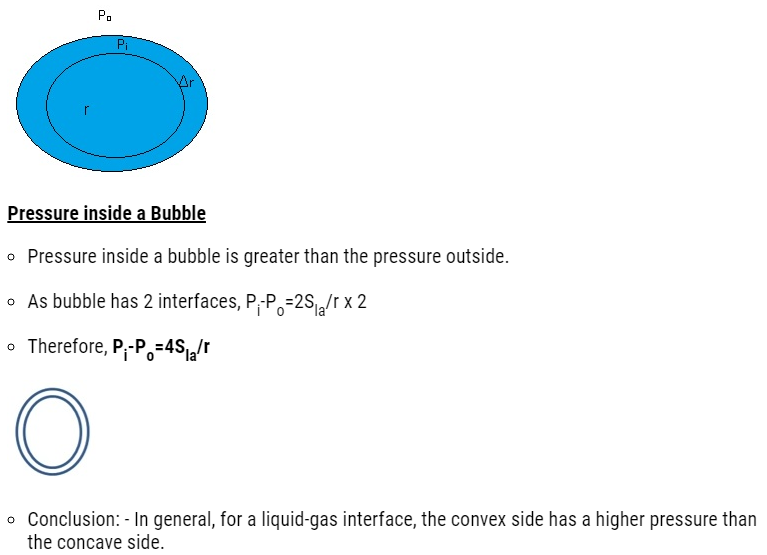
Pressure inside a drop and a cavity
- Pressure inside a drop is greater than the pressure outside.
- Suppose there is a spherical drop of water of radius ‘r’ which is in equilibrium.
- Consider there is increase in radius which is Δr.
- Therefore Extra Surface energy = Surface Tension(S) x area
- = Sla x 4π(r+Δr)2 – Slax4πr2
- After calculating
- Extra Surface energy=8πr Δr Sla
- At Equilibrium, Extra Surface energy = Energy gain due to the pressure difference
- 8πr Δr Sla = (Pi – Po) 4πr2xΔr
where Pi= Pressure inside the drop and Po = Pressure outside the drop.
After calculation Pi – Po = 2 Sla/r
Capillary Rise
- In Latin the word capilla means hair.
- Due to the pressure difference across a curved liquid-air interface the water rises up in a narrow tube in spite of gravity.
- Consider a vertical capillary tube of circular cross section (radius a) inserted into an open vessel of water.
- The contact angle between water and glass is acute. Thus the surface of water in the capillary is concave. As a result there is a pressure difference between the two sides of the top surface. This is given by
- (Pi – Po) =(2S/r) = 2S/(a sec θ )= (2S/a) cos θ (i)
- Thus the pressure of the water inside thetube, just at the meniscus (air-water interface)is less than the atmospheric pressure.
- Considerthe two points A and B. Theymust be at the same pressure,
- P0 + h ρ g = Pi = PA (ii)
- where ρ is the density of water,and h is called the capillary
- h ρ g = (Pi – P0) = (2S cos θ )/a (By using equations (i) and (ii))
- Therefore the capillary rise is due to surface tension. It is larger, for a smaller radius.
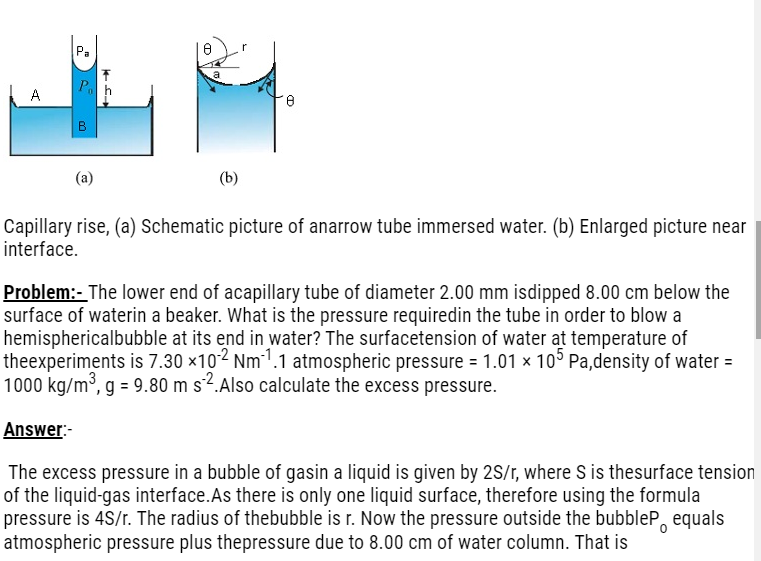
Po = (1.01 × 105 Pa + 0.08 m × 1000 kg m–3× 9.80 m s–2)
= 1.01784 × 105 Pa
Therefore, the pressure inside the bubble is
Pi = Po + 2S/r
= 1.01784 × 105 Pa + (2 × 7.3 × 10-2 Pa m/10-3 m)
= (1.01784 + 0.00146) × 105 Pa
= 1.02 × 105 Pawhere the radius of the bubble is taken to be equal to the radius of the capillary tube, since the bubble is hemispherical!